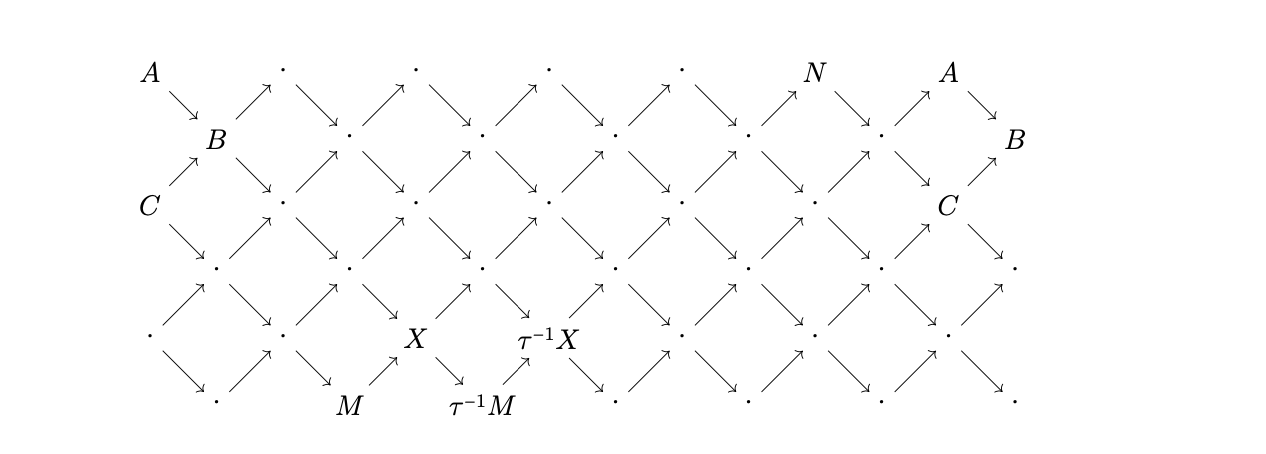
I have a question about proving that a module is non-rigid using Auslander-Reiten quiver. Suppose that we have some algebra $A$ and the components of its Auslander-Reiten quivers are tubes like the following picture.
Call the number of modules in the first row the rank of the tube. In the tube of the picture above, the rank is $6$.
I heard from a professor that the modules in $i$th rows are non-rigid $i \ge 6$. But I cannot find a proof of this when I search on google. Are there some literature about this fact?
I am trying to prove that the module $M$ on the $6$th row is non-rigid as follows. By Auslander-Reiten formula, \begin{align*} {\rm Ext}^1(M,M) \cong D \underline{{\rm Hom}}(\tau^{-1}M, M). \end{align*}
So it suffices to find a non-trivial map $\tau^{-1}M \to M$. Using the picture, we see that there is a map from $\tau^{-1}M$ to $N$, there is a map from $N$ to $C$, and there is map from $C$ to $M$. Therefore we have a map $\tau^{-1}M \to M$.
But I am a bit confused. If we consider the module $X$ on the $5$th row of the tube, we have maps $\tau^{-1}X \to N$, $N \to C$, $C \to M$, $M \to X$. Therefore we also have a map from $\tau^{-1}X$ to $X$. Then $X$ would be non-rigid. But this is not true. It is possible that $X$ is rigid since $X$ is on the $5$th row which is less than the rank $6$. What is the difference between $X$ and $M$? Thank you very much.