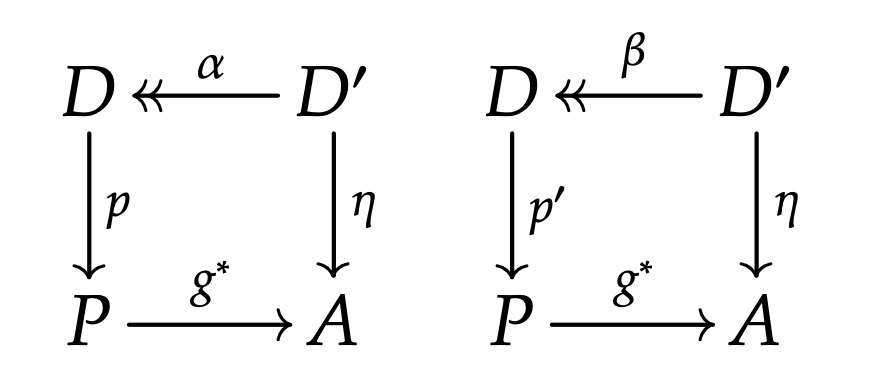Claim: Consider a category, such that for any two objects $X,Y$ there exists an object $Z$ and epimorphisms $Z\twoheadrightarrow X$ and $Z\twoheadrightarrow Y$. We call such a $Z$ and the epis a common cover of $X$ and $Y$.
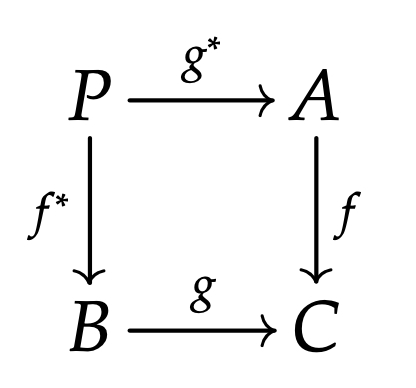
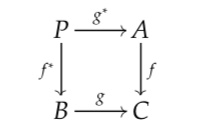
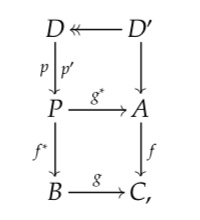
Let
be a Cartesian square. We shall write $[p]$ for a generalized element with representative $p$. Let $[p],[p']$ be two generalised elements of $P$ such that $f^*([p])=f^*([p'])$ and $g^*([p])=g^*([p'])$. Then $[p]=[p']$.
Proof: Replacing the domains of $p$ and $p'$ with a common cover, we can assume, that they have the same domain $D$. The equation $f^*(p)=f^*(p')$$g^*(p)=g^*(p')$ means that we have two commutative diagrams
withe the same $eta$. We can replace $D$ by $D'$ and get a diagram
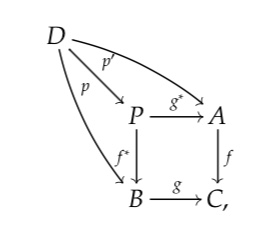
which commutes for $p$ and for $p'$ separately. In the same way we get an arrow $D\to B$ such that the diagram
which commutes for $p$ and for $p'$. But now the uniqueness in the definition of a Cartesian square yields $p=p'$.