To place the formula of the OP into context, it is helpful to note the identity (from Josefsson - More characterizations of cyclic quadrilaterals)
$$\frac{q-p}{q+p}=\frac{(a-c)(b-d)}{(a+c)(b+d)}.\tag{$*$}\label{star}$$ Here $q$ and $p$ are the lengths of the blue and red diagonals, with opposite angles $\alpha$ and $\beta$.

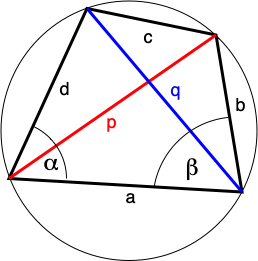
The quadrilateral tangent formula can thus be rewritten in a form that looks more like the triangle tangent formula, $$\frac{\tan\frac12(\alpha-\beta)}{\tan\frac12(\alpha+\beta)}=\frac{q-p}{q+p}.$$
Q: Is it obvious that the law of tangents applies to the blue and red diagonals?
The formula \eqref{star} is equivalent (upon rearrangement of the terms) to what is known as Ptolemy’s second theorem $$\frac{p}{q}=\frac{ad+bc}{ab+cd}.$$
