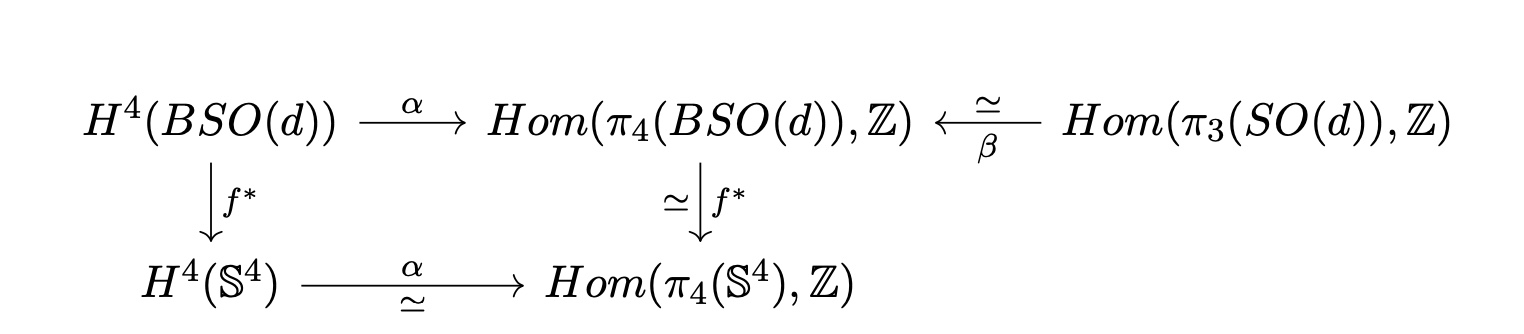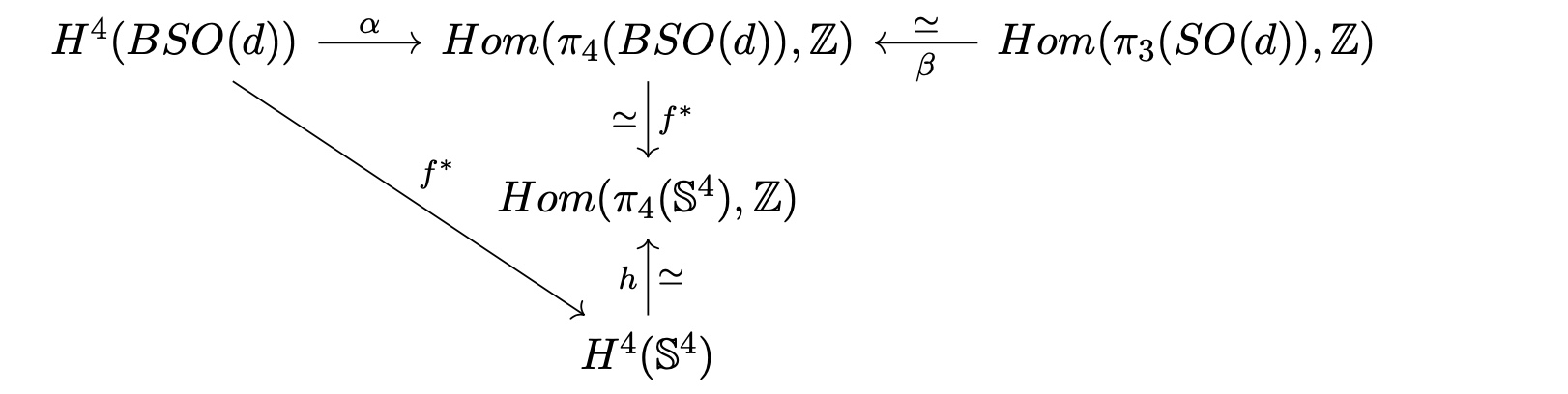Let me try to summarize various observations from the comments and put it all together.
Let $d\ge 5$ and let $f: S^4\to BSO(d)$ be a generator of $\pi_4(BSO(d))\cong \mathbb Z$.
Let $\gamma^d$ be the universal orientable $\mathbb R^d$ vector bundle over $BSO(d)$.
Then we have the following diagram extending the one by the OP. It is easily seen to commute by naturality.
Note that as indicated on the diagram the bottom $\alpha$ and the right $f^*$ are isomormphisms.
Observe that $f^*(p_1(\gamma^d))=p_1(f^*(\gamma^d))$. It is well known that $p_1$ of any vector bundle over $S^4$ is divisible by 2 (if we identify $H^4(\mathbb S^4)$ with $\mathbb Z$) and in fact every even number is realized. In particular $p_1$ of the Hopf $\mathbb R^4$ bundle is $\pm 2$. The above is done in detail for example in Milnor's classical paper on exotic $7$-spheres.
Since the two arrows ending in $Hom(\pi_4(\mathbb S^4), \mathbb Z)$ are isomorphisms this implies that the image of the top row $\alpha$ is exactly $2\mathbb Z$ (we again identify $Hom(\pi_4(BSO(d)),\mathbb Z)$ with $\mathbb Z$).
Moreover this also shows that $\alpha(p_1)=\pm 2\beta(x)$ (recall that $x\in Hom(\pi_3(SO(d),\mathbb Z)$ is a generator).
Furthermore this shows that the clutching map of the stabilized Hopf bundle is exactly the generator of $\pi_3(SO(d))$ since if it wasn't then $p_1$ of the Hopf bundle would be a nontrivial multiple of $2$.
The clutching map of the Hopf bundle is just the identity map $\mathbb S^3=Sp(1)\to Sp(1)\cong SU(2)\subset SO(4)$ and therefore the generator of $\pi_3(SO(d))$ comes from the inclusion $SU(2)\to SO(4)\to SO(d)$.
This can also be seen in other ways without using Pontryagin classes. For example, recall the following well known picture of $SO(4)$.
Think of $\mathbb R^4$ as quaternions and $\mathbb S^3$ as $Sp(1)$ then consider the ineffective isometric linear $\mathbb S^3\times \mathbb S^3$ action on $\mathbb R^4$ given by $(q_1,q_2)(v)=q_1vq_2^{-1}$. The kernel is $\mathbb Z_2=\{\pm (1,1)\}$ and we get $SO(4)=(\mathbb S^3\times \mathbb S^3)/\mathbb Z_2$. The first $\mathbb S^3$ commutes with multiplication by $i$ on the right and the second with multiplication by $i$ on the left. This identifies the two $Sp(1)$'s with two different embeddings of $SU(2)$ in $SO(4)$. The right $Sp(1)$ is easily seen to correspond to the standard embedding. The two $SU(2)$' give generators of $\pi_3(SO(4))\cong\mathbb Z\oplus \mathbb Z$. Now look at the inclusion $SO(4)\to SO(5)$ and the induced map on $\pi_3$. From the homotopy sequence of the bundle $SO(4)\to SO(5)\to S^4$ we get that the map $\pi_3(SO(4))\to \pi_3(SO(5))\cong \mathbb Z$ is onto.
Since there is a unique irreducible real representation of $SU(2)$ of dimension 4 the two $SU(2)$'s are conjugate in $O(4)$ (but of course not in $SO(4)$). This conjugation can be made explicit by observing that $\overline{q(\bar v)}=v\bar q=vq^{-1}$. Hence quaternionic conjugation written as an element of $O(4)$ conjugates the two $SU(2)$'s. Under the standard identification of $\mathbb R^4$ with $\mathbb H$ given by $(a,b,c,d)\mapsto a+bi+cj+dk$ the conjugating matrix is diagonal $diag(1,-1,-1,-1)$.
The two $SU(2)$'s become conjugate in $SO(5)$ by $diag(1,-1,-1,-1, -1)$. Hence the $SU(2)$'s have the same image in $\pi_3(SO(5))$ which must be the generator else $\pi_3(SO(4))\to \pi_3(SO(5))$ would not be onto.
Lastly, this could be seen using general theory by computing the Dynkin index of $SU(2)$ in $SO(5)$. Given a simple compact Lie group $G$ and its simple subgroup $H$ normalize their Killing forms $(\cdot, \cdot)_G$ and $(\cdot, \cdot)_H$ so that the longest roots have length $\sqrt 2$. Then the restriction of $(\cdot, \cdot)_G$ to the Lie algebra of $H$ is proportional to $(\cdot, \cdot)_H$. The coefficient is called the Dynkin index of $H$ in $G$. It is always an integer and up to sign is equal to the coefficient in the induced map $\pi_3(H)\cong \mathbb Z\to \pi_3(G)\cong \mathbb Z$. For the proof see the book "Topology of transitive transformation groups" by Onishchik.
It is not hard to compute that the Dynkin index of $SU(2)$ in $SO(5)$ is 1 but won't do it as we have two other proofs already.