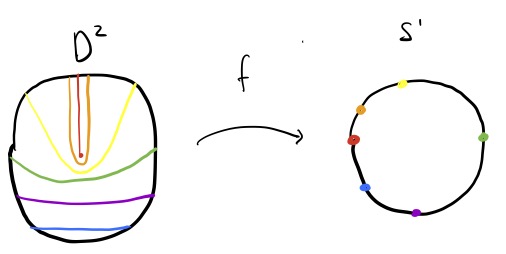
I think you can do something like this. Let $Y=D^2$ be the open disc in the plane, $X=S^1$ the circle. Let $X_1$ a point on the circle (the red point below) and $X_2=X\setminus X_1$. Define the map as in the picture below. The fiber over the red point is the red half line $\{0\}\times [0,1)$. The fibers over the other points are diffeomorphic to open intervals. The map does not induce an isomorphism in homology, but it does do so when restricted over the preimages of $X_1$ and $X_2$.
Edit: The question was changed after this answer