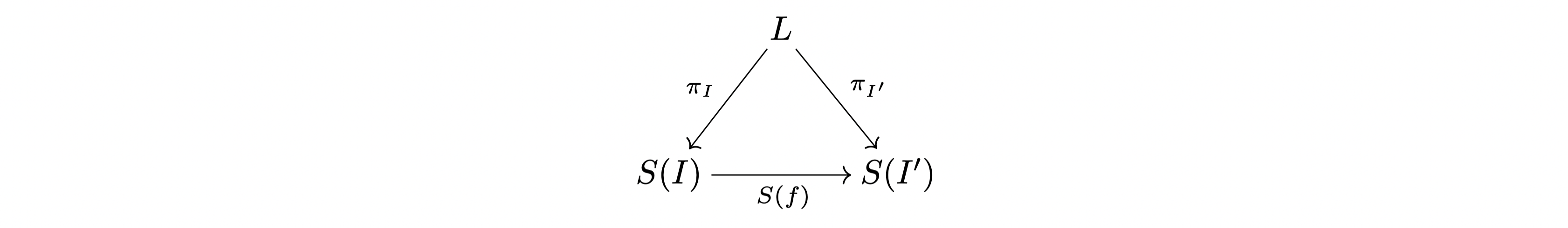Proof Let $F:\mathcal{C}\simeq\mathcal{D}$ be an equivalence with $S:\mathcal{I}\to\mathcal{C}$ be a diagram of shape $\mathcal{I}$ in $\mathcal{C}$, and suppose that $F\circ S$ has a limit $\pi':\Delta L'\Rightarrow F\circ S$. Since $F$ is essentially surjective there exists some object $L\in\mathcal{C}$ and an isomorphism $u:F(L)\cong L'$, so $\pi'\circ u:\Delta F(L)\Rightarrow F\circ S$ is also trivially a limit of $F\circ S$. Further, since $F$ is full we obtain a source $$\hat L=\{\pi_I:L\to S(I)\}_{I\in\mathcal{I}}$$ in $\mathcal{C}$ with $F(\pi_I)=\pi'_I\circ u$ for all objects $I\in\mathcal{I}$, thus $F(\hat L)\cong\pi'$ as cones since $u$ was an iso and a morphism of cones by the preceding equations for all $I$. If any other source $\hat L''=\{\pi'':L''\to S(I)\}_{I\in\mathcal{I}}$ satisfies $F(\hat L'')\cong\pi'\cong F(\hat L)$ then there exists a unique isomorphism of cones $$w':F(L)\to F(L''),$$ and since $F$ is full this arrow is the image of an arrow $w:L\to L''$ which is unique satisfying $F(w:L\to L'')=w':F(L)\to F(L'')$ by faithfulness of $F$. We then have that $$F(\pi''_I\circ w)=F(\pi''_I)\circ F(w)=F(\pi''_I)\circ w'=F(\pi_I)\implies\pi''_I\circ w=\pi_I,$$ since $F$ is faithful, and $w$ is an iso since $w'$ is with $w^{-1}:L''\to L$ the unique arrow such that $F(w^{-1}:L''\to L)=w'^{-1}:F(L'')\to F(L)$, so $\hat L''\cong\hat L$ and since $\hat L''$ was an arbitrary source $\hat L$ is the unique source up to isomorphism satisfying $F(\hat L)\cong\pi'$. We further have that $\pi:\Delta L\Rightarrow S$ is a limit of $S$; it is a cone since
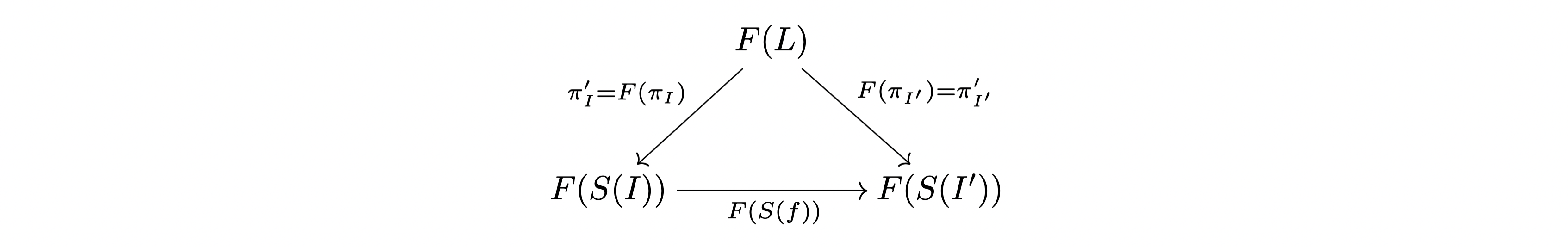
commutes for all arrows $f:I\to I'\in\mathcal{I}$ since $\pi'$ is a cone to $F\circ S$, thus
commutes for all arrows $f:I\to I'\in\mathcal{I}$ since $F$ is faithful. This cone is further terminal, since any other cone $'\pi:\Delta'L\Rightarrow S$ gives rise to a cone $F('\pi):\Delta F('L)\Rightarrow F\circ S$ which induces a unique morphism of cones $'u:F('L)\to F(L)=L'$ with $F(\pi_I)\circ{'u}=\pi'_I\circ {'u}=F({'\pi_I})$, and since $F$ is full there exists an arrow $v:{'L}\to L\in\mathcal{C}$ which is unique satisfying $F(v:{'L}\to L)={'u}:F({'L})\to F(L)$ since $F$ is faithful, and $v$ is also a morphism of cones since $$F(\pi_I\circ v)=F(\pi_I)\circ F(v)=\pi'_I\circ{'u}=F({'\pi_I})\implies\pi_I\circ v={'\pi_I}$$ for all objects $I\in\mathcal{I}$, again by faithfulness of $F$.