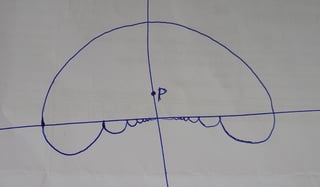
The result is not necessarily true, consider. Consider for $n\geq1$ the circumferencesopen ball $S_n$ such that$R_n$ with diameter from $(\frac{1}{n},0)$ andto $(\frac{1}{n+1},0)$ form a diameter of $S_n$, and let. Let $L_n$ be $S_n\cap \{y\leq0\}$ and $R_n$ be the reflection of $L_n$$R_n$ with respect to the $y$ axis.
Now considerlet $A$ be the open set bounded byunion of the $L_n$, the $R_n$ and the upper semicircunferencehalf of center $0$ and radius $1$the open unit disc. Then the function $x\mapsto m(x)$ is not continuous at $p=(0,0.1)$. This is because $m(p)=(0,0.5)$, but for the sequence of points $p_n=(\frac{1}{2}(\frac{1}{n}+\frac{1}{n+1}),0.1)$, we have $m(p_n)=p_n$ for $n\geq2$.