This is a follow-up to John's answer.
Here is the questionable "theorem" from the 3rd2nd (2013) edition of Erickson's book (thanks @spin for the pointer), which in the 2nd1st (1996) edition was numbered as Theorem 9.3.
Apparently, the statement about "only two feasible sets of parameters for perfect codes" is correct, but "theorem" as stated lacks a proof (and thus may be incorrect) and was not an argument for non-existence of other perfect codes. Reference [22] is Pless' book. 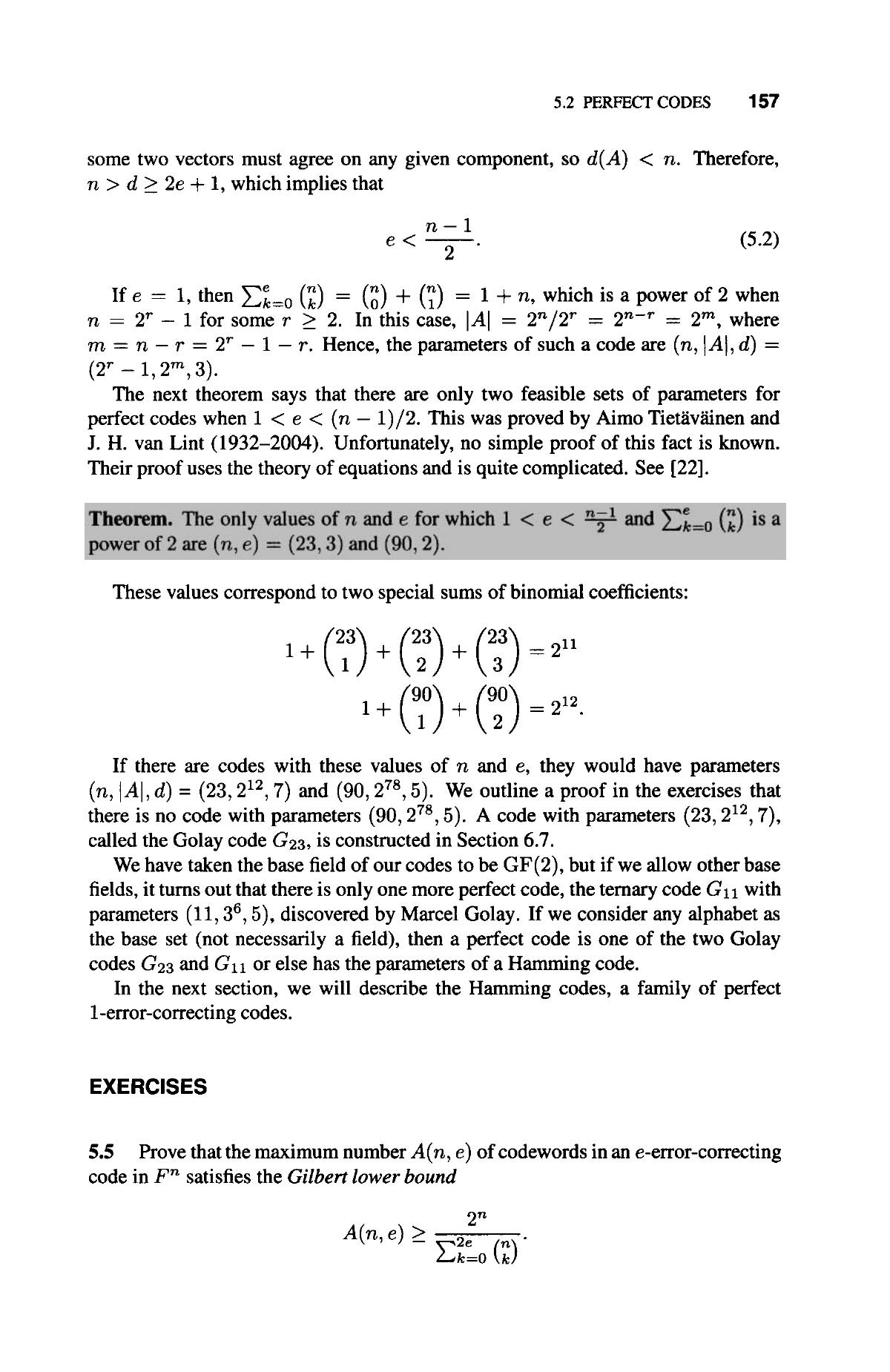
And here are two pages (pages 23-24) with a relevant discussion from the 3rd (1998) edition of Pless' book. It does mention Tietäväinen and van Lint results on page 24, but they do not imply the "theorem" from Erickson's book. Reference [29] is
A. Tietäväinen, "On the nonexistence of perfect codes over finite fields", SIAM J. Appl. Math. 24 (1973), 88-96.



