I accidently found a class of rank-deficient square matrices that are their own Moore-Penrose pseudo inverse:
$$\boldsymbol{A}\in\mathbb{R}^{n\times n}: a_{(i,i)}=n-1,\, a_{\lbrace i,j\rbrace}=-1\implies\Big(\frac{1}{n}\boldsymbol{A}\Big)^+ =\Big(\frac{1}{n}\boldsymbol{A}\Big)$$
as an exemplary $4\times 4$ calculation done with WA demonstrates
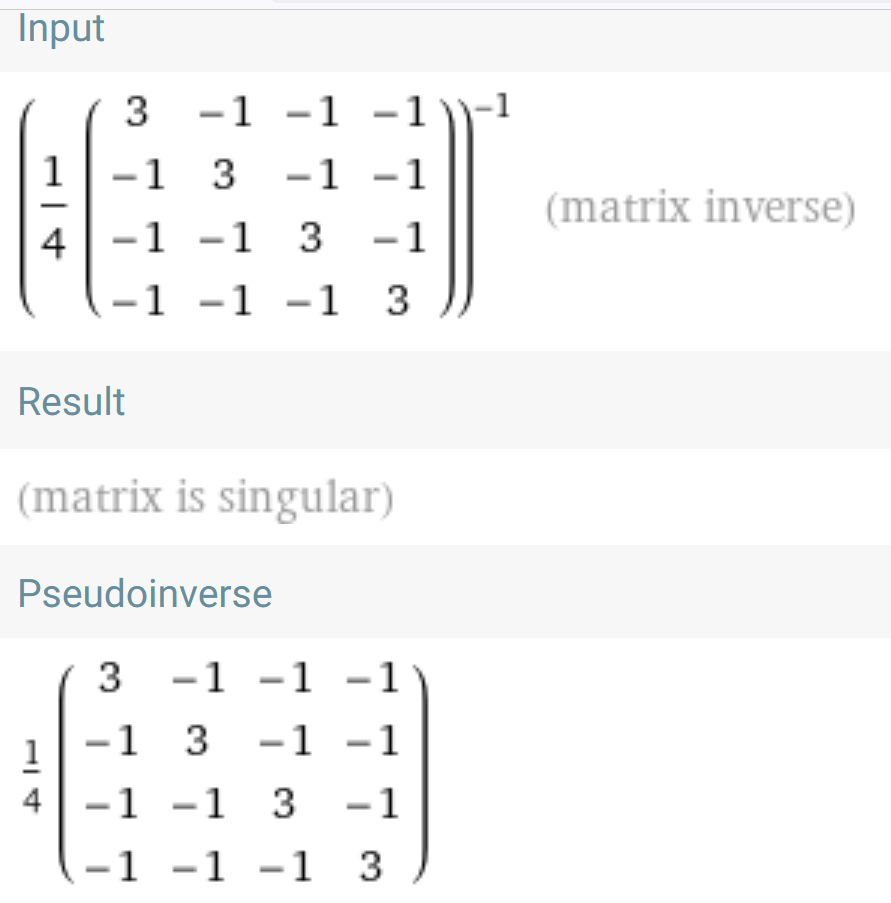
Questions:
- have all Moore-Penrose "pseudo unit-matrices" been characterized, what about other kinds of pseudo inverses?
- do these "pseudo unit-matrices" play a special role in the theory of pseudo inverses, resp., in linear algebra?
