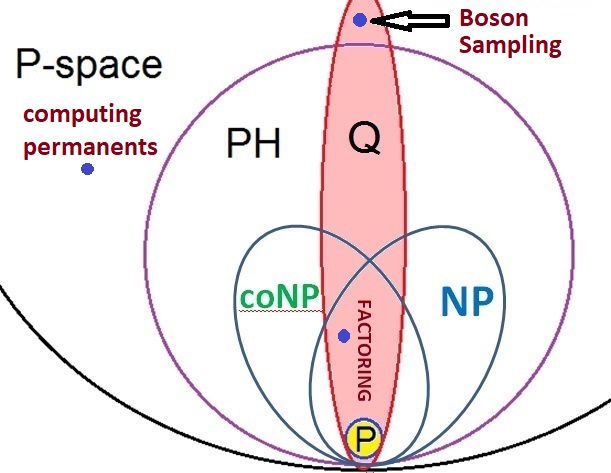
In addition to Shor's algorithm and to Grover algorithm let me mention two important pieces of information that abstract quantum computer have for some specific goals superior computational powers.
The ability of quantum computers, and even quantum circuits of bounded depth to perform sampling tasks that goes beyond the polynomial hierarchy! (Evidence that ${\bf QuantumSampling \not \subset PH}$.)
The evidence that the class of decision problems that quantum computers can solve goes beyond the polynomial hierarchy! (Evidence that ${\bf BQP \not \subset PH}$)
The first item is a long story related to early works of Terhal and DiVincenzo (2004), Aaronson and Arkhipov (2013) and Bremner, Jozsa, and Shepherd (2011). Related also to Boson Sampling.
The second item (with a weaker type of evidence) is related to a 2018 work by Raz and Tal.
${\bf QuantumSampling}$ (which is one aspect of the power of quantum computers that reflects the power of quantum computers for sampling) may be in a sense stronger than ${\bf BQP}$ (that reflects theThe power of quantum computers for samplingdecision problems) since it is a plausible conjecture that ${\bf QuantumSampling \not \subset P^{BQP}}$. (And even ${\bf QuantumSampling} \not \subset {\bf PH^{BQP}}$.)