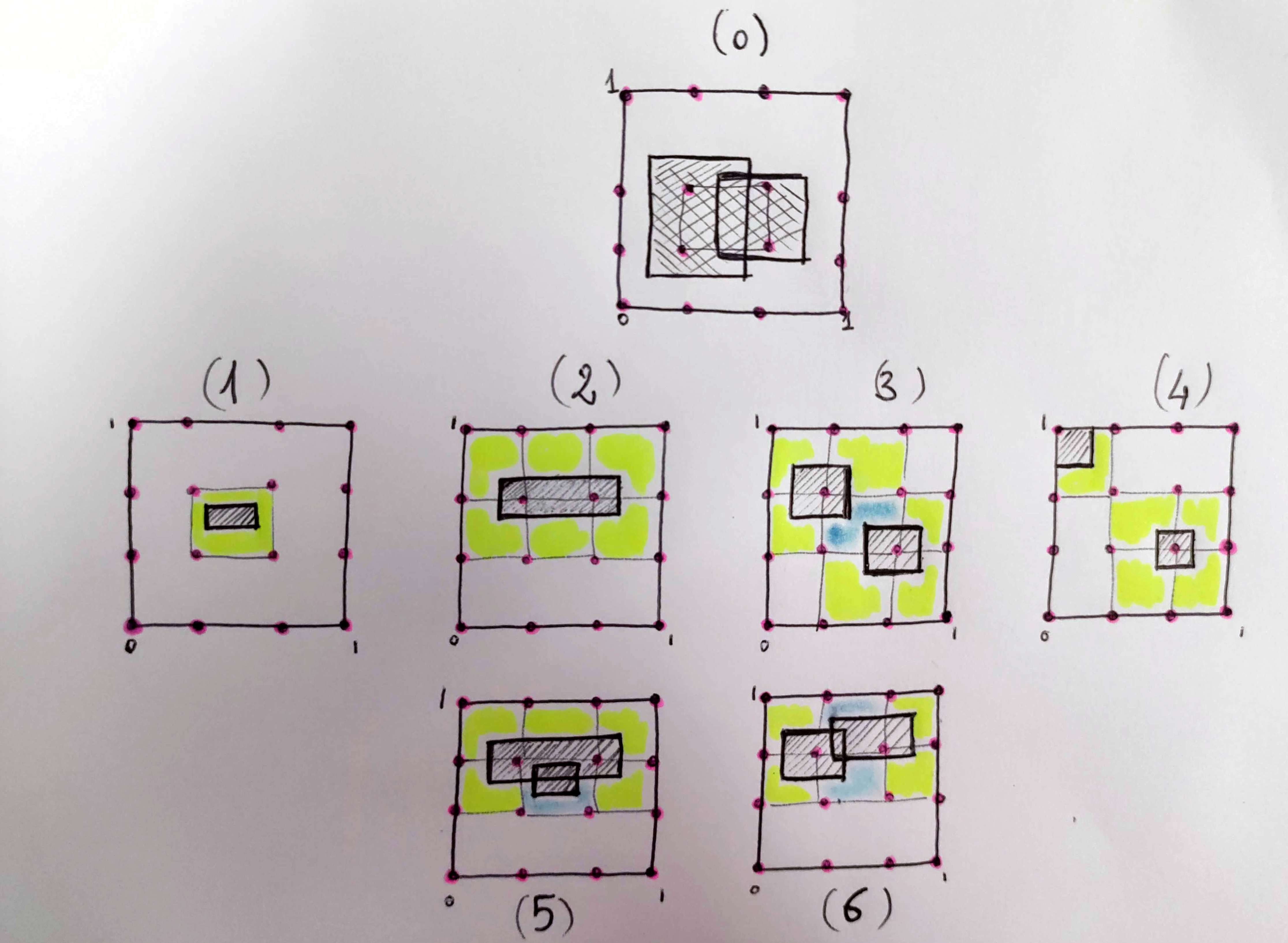
I'm reporting here some questions on the answer below. In this picture I consider 67 possible cases. Each quadrant is $[0,1]^2$. The pink dots are the elements of $\mathcal{S}\equiv \mathcal{S}_1\times \mathcal{S}_2$ (recall that in my simplified setting $\mathcal{S}$ is finite).
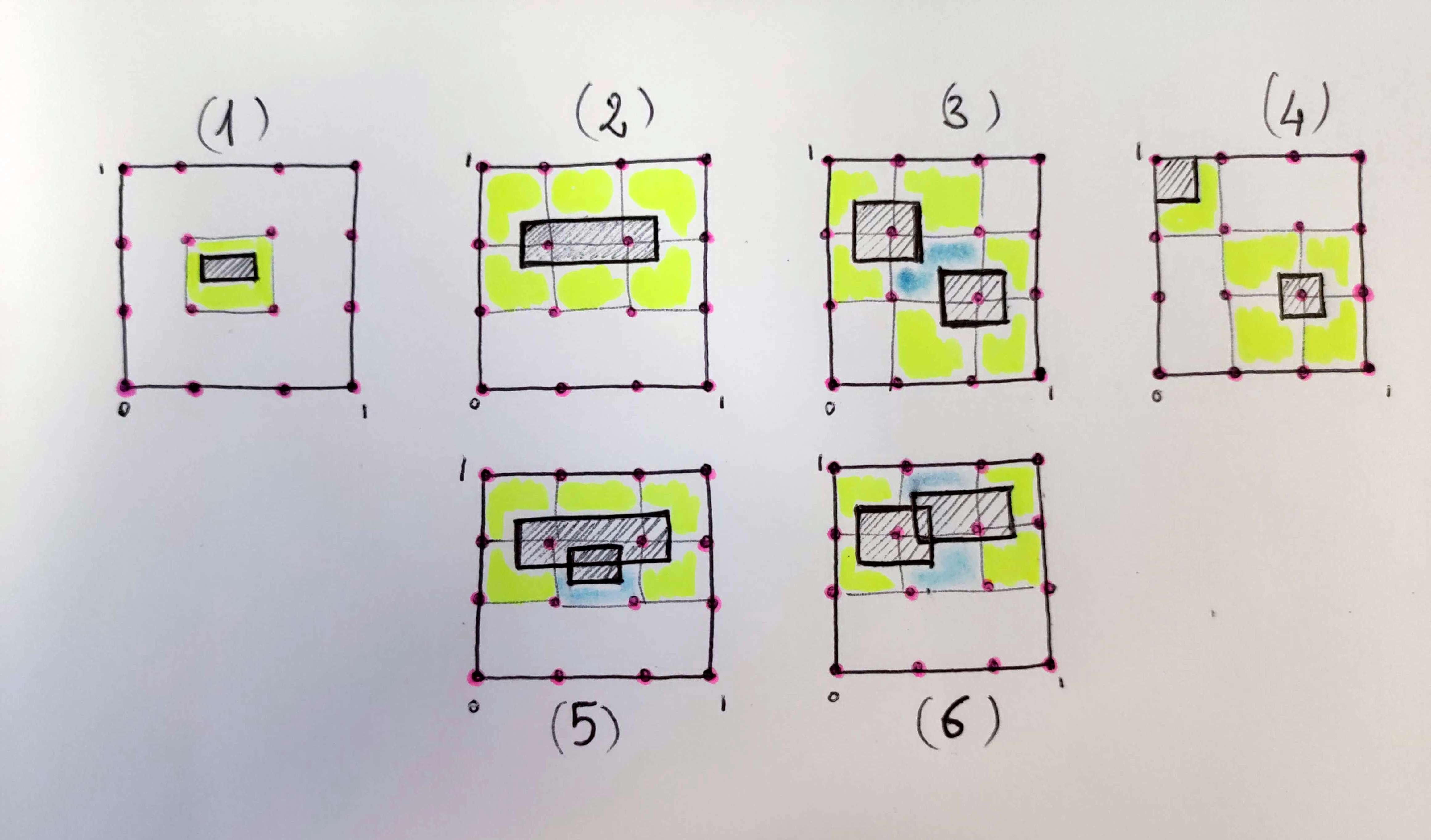
Case 4 seems similar to cases 1,2.
I have one question at this stage: (Q1) Why the shift of the mass should be "uniform"?
Let us now move to the pathological cases which, if I have understood correctly your answer, are cases 3,5, and 6. Consider, for instance, now case 3. Here there are two boxes, $B_1,B_2$, whose volume we want to be zero. Associated with box $B_1$, there are 4 atomic boxes, $\{D_{i,1}\}_{i=1}^4$, from the sub-copula. Associated with box $B_2$, there are also 4 atomic boxes, $\{D_{i,2}\}_{i=1}^4$, from the sub-copula. $B_1$ and $B_2$ share one atomic box. This means that the blue region should accommodate part of the mass coming from both $B_1$ and $B_2$. The answer below suggests that this creates issues in the "mass-redistribution" procedure
Cases 5 and 5 seems similar to case 3. My second question is: (Q2) Why
Now, let's move to the problematic case, which is case 0 on top. In this case an issue? Can I read this as saying "there cannot beatomic box is contained in the union of the two shaded boxes $B_1,B_2$, whose. Since such atomic box may not have volume we wantequal to be zero$0$ under $\bar{C}$, that have an atomic box in common"?then when swapping masses we violate the "extension" feature.
To sum upQ1: I'm not sure about when/whyIs the "mass-redistribution" procedure fails inabove interpretation of the answer correct?
Q2: Suppose I can exclude the problematic case where two or more boxes are required to have zero volume0. In particularThen, I thinkcan we formally show that since $\mathcal{S}$ is finite in my case, there shouldthe swapping of masses can be no issue.done in all the 6 cases above? How?