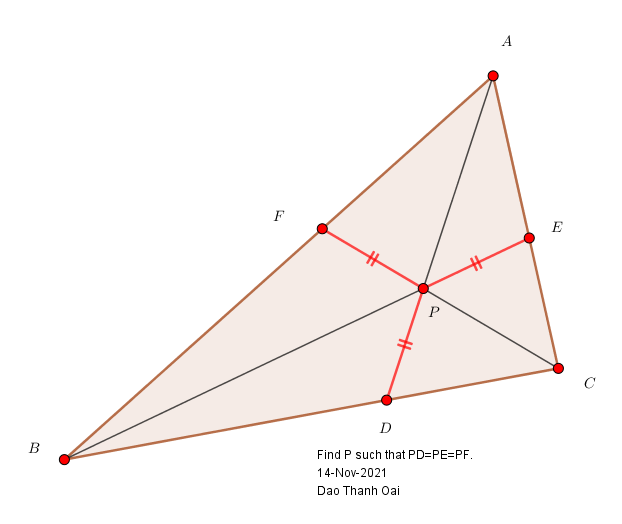
Some years ago, I asked some 'famous' people in an advanced Plane Geometry forum about the following:
Let $ABC$ be arbitrary triangle, how can one construct a point $P$ in the plane such that $P$ is the circumcenter of the cevian triangle of $P$ with respect to $ABC?$
The answer was negative, even in the sense of calculations by a Computer (can't construct by rule and compass, even can not calculations by computer).
My conjecture: Let $ABC$ be arbitrary triangle, then there exist a point $P$ such that $P$ is the circumcenter of the cevian triangle of $P$ with respect to $ABC$.
Question: How can prove conjecture and how construct this point?
I propose this conjecture to you for a clear proof.