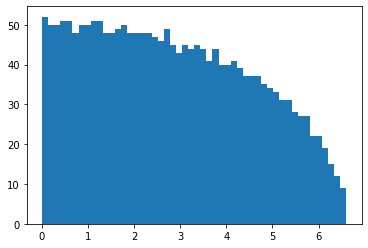
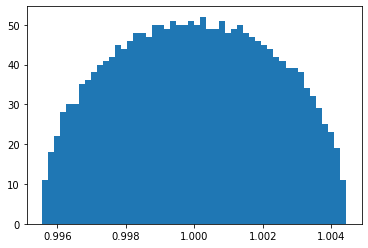
Let $A\in\mathbb R^{n\times n}$ be a random Gaussian matrix with i.i.d entries from $\mathcal N (0, \frac{a}{\sqrt{n}})$. By Marchenko-Pastur we know the limiting distribution of the eigenvalue of $A^TA$. Now I want to understand the eigenvalue behavior of $(I-A)^{T}(I-A)$. Based on some simulations, if $a$ is big, then the distribution converges to the quarter circle law (which is intuitive as the effect of $I$ shrinks), and a small $a$ leads to a deformed semicircle. Is there an analytical depiction of this distribution? Thanks.
Bumped by Community user