Not sure why my answer received negative votes. I think it's correct. Someone please point out my errors?
EDITED TO REFLECT @LUCIA'S COMMENTS: After digging through a bunch of references, I sorted out the answer I was looking for. For all $T > 0$ except at the points of discontinuity of $N(2\pi T)$, one has $$N(2 \pi T) =1+ \frac{1}{\pi} \theta(2\pi T) + \frac{1}{\pi}\operatorname{Arg}\zeta\left(\frac{1}{2}+2\pi i T\right)+2R(T),$$ where $\operatorname{Arg}$ is the principal branch of the argument, where $\theta(T)$ is the Riemann-Siegel theta function, and where $R(T)$ is an integer that is $O(\log T)$. According to p. 98 of Davenport's book, you can get rid of the $R(T)$ integer term by replacing the principal value of $\arg \zeta(1/2+2 \pi i T)$ with the variation of $\arg \zeta(s)$ from $s = +\infty+2\pi iT$ to $1/2+2 \pi iT$ starting with value $0$, as long as $T$ is not $\frac{1}{2\pi}$ times an ordinate of a zero of $\zeta(s)$.
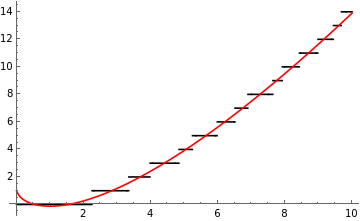
By a known asymptotic expansion of $\theta(t)$, one has the asymptotic relation \begin{align*} N(2 \pi T) & =T \log T-T+\frac{7}{8}+\frac{1}{\pi}\operatorname{Arg}\zeta\left(\frac{1}{2}+2\pi i T\right)+2R(T)+O\left(\frac{1}{T}\right) \\ & = \int_1^T \log t \, dt -\frac{1}{8}+\frac{1}{\pi}\operatorname{Arg}\zeta\left(\frac{1}{2}+2\pi i T\right)+2R(T)+O\left(\frac{1}{T}\right) \end{align*} and the asymptotic expansion \begin{align*} N(2 \pi T) - \frac{1}{\pi}\operatorname{Arg}\zeta\left(\frac{1}{2}+2\pi i T\right) \sim T \log T - T+ 2R(T) + \frac{7}{8}+\frac{1}{96 \pi^2 T}+ \frac{7}{11340\pi^4 T^3}+ \frac{31}{161280 \pi^6 T^5}+\cdots \end{align*} as $T \to \infty$, where the numerators and (1/2)denominators are as in OEIS Sequences A036282 and A114721, respectively. Here is a plot of the function $N(2\pi T)$ and its smooth approximation $1+\frac{1}{\pi}\theta(2\pi T)$.
What is the infimum of all $T$ that is not $\frac{1}{2\pi}$ of an imaginary part of a zero of $\zeta(s)$ such that $R(T)\neq 0$? Table 2 of Bober's and Hiary's "New computations of the Riemann zeta function on the critical line" (Exp. Math. 27 (2) (2018) 125--137) implies that the infimum is $\leq 7757304990367861417150213053.638\ldots$, though their methods don't rule out a tighter upper bound. I think this is an important question that has not been addressed yet.