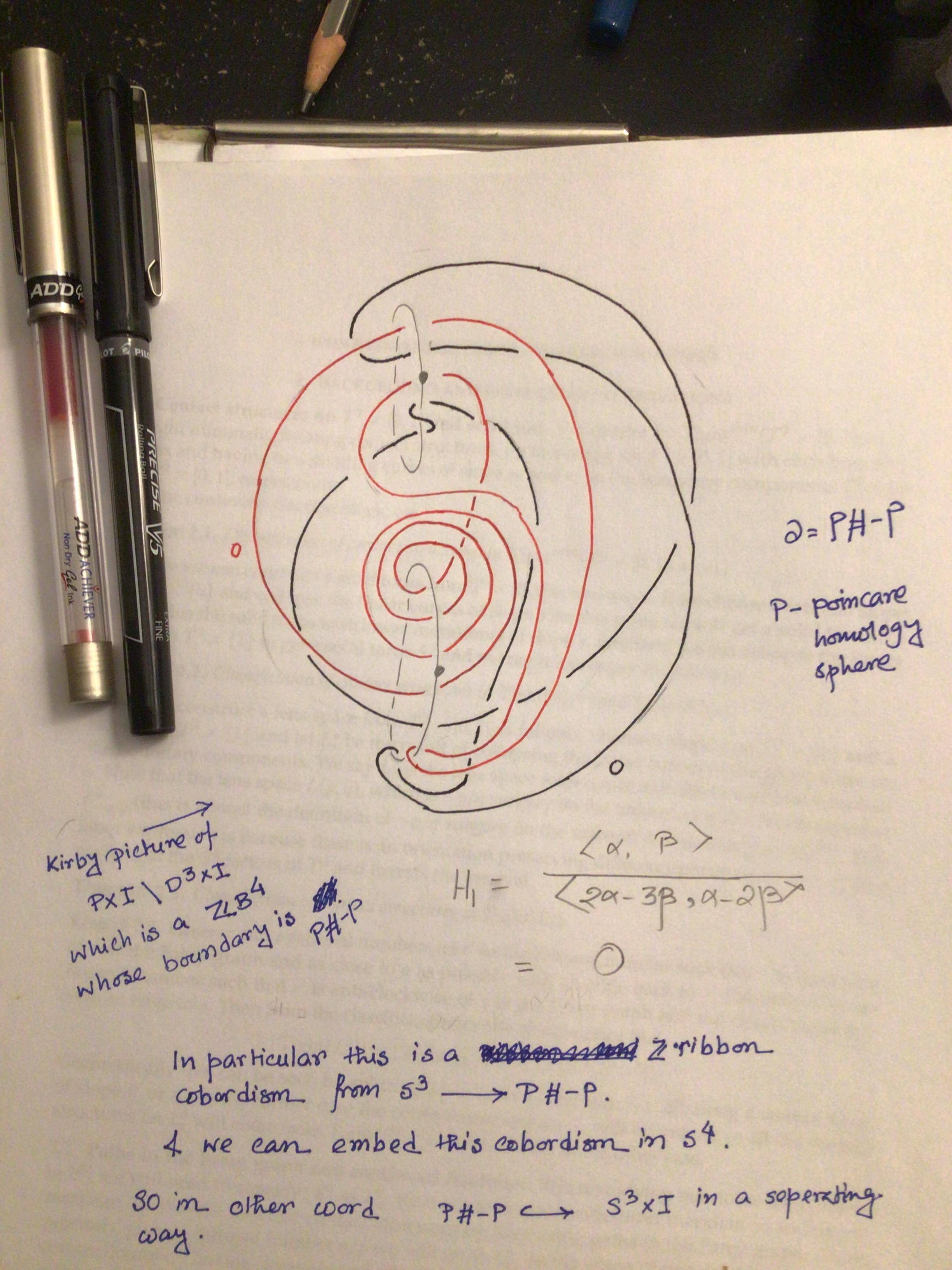
Yes, such domain exists. Let $P$ be Poincare homology 3-sphere. And $X= P\times I - D^3\times I$, then X$X$ can be smoothly embedded in $S^4$(mostly the double of $X$ is $S^4$) . Let $\Omega$ be a small open neighbourhood of $X$. Then notice that $\Omega$ deformation retract onto $X$, but $\Omega$ cannot be contractible as $X$ is not.
There is a deeper reason why a homology ball that $P\# -P$ bounds cannot be contractible, and it is essentially follows from Taube's Periodic Endperiodic end theorem.
Here is one of my attempt to draw a picture.