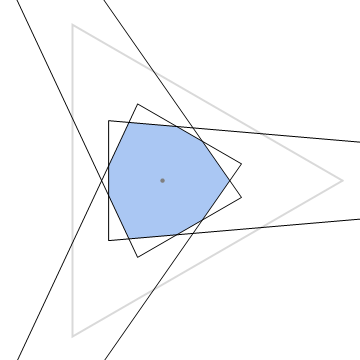
I find an area of $.122732$$.245463$ for the intersection when each $T_i$ has a vertex at distance $2.23329$$3.15835$ from the center of $T$. This seems to be maximal among the symmetric options.
The gray triangle in the diagram is $T$, the black triangles are the $T_i$ (with their specified far vertices outside the area of the diagram), and the blue nonagon is the desired intersection.
If the distance is $(1+2b)R$, where $R$ is the circumradius of $T$, we can write the area exactly as $$4\left(2b - b^2 - \frac{3}{4b^2-1} - \frac{8b^2-4b-2}{12b^2 -1}\right)$$
which is easy to maximize numerically.
For a quicker approximate answer, we can assume that the area of intersection is a circle, tangent to the two long sides of $T_1$ at $(r,\theta)$ in polar coordinates. Then we can solve for $r,R,\theta$ satisfying \begin{align} 3R^2 \sqrt{3} / 4 &= \,1, \text{ for circumradius of }T;\\ r(\sec \theta - 2)/3 &= R, \text{ for centroid of }T_1;\\ r^2(1+\sec \theta)^2 \cot \theta &= \,1, \text{ for area of }T_1 \end{align} and the area and distance quoted above are $\pi r^2\simeq .225$ and $r \sec \theta \simeq 3.17$.