Here is a plot of the average of $|{\rm Tr}\,U|$ over ${\rm U}(n)$ as a function of the matrix size $n/10$ (averaged over $4\cdot10^3$ random matrices). The horizontal line is the large-$n$ asymptote noted by Ofir Gorodetsky, $$\int_{{\rm U}(n)} |\,{\rm Tr}\,(U)|\,dU\rightarrow \frac{1}{\pi}\int_{-\infty}^\infty dx\int_{-\infty}^\infty dy\,\sqrt{x^2+y^2} e^{-x^2-y^2}= \tfrac{1}{2}\sqrt\pi,$$ which is reached within a fewone percent for $n\gtrsim 10$.
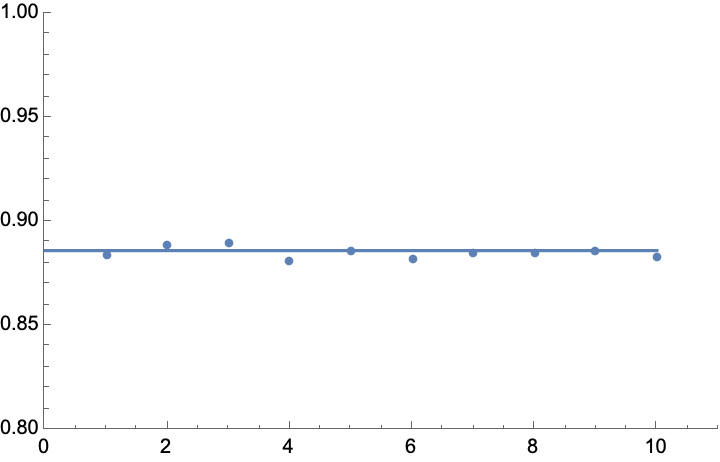
horizontal axis is matrix size $n$ divided by 10
