A$\newcommand{\la}[1]{\kern-1.5ex\leftarrow\phantom{}\kern-1.5ex}\newcommand{\ra}[1]{\kern-1.5ex\xrightarrow{\ \ #1\ \ }\phantom{}\kern-1.5ex}\newcommand{\ras}[1]{\kern-1.5ex\xrightarrow{\ \ \smash{#1}\ \ }\phantom{}\kern-1.5ex} \newcommand{\ua}[1]{\bigg\uparrow\raise.5ex\rlap{\scriptstyle#1}}\newcommand{\da}[1]{\bigg\downarrow\raise.5ex\rlap{\scriptstyle#1}}\DeclareMathOperator{\sgn}{\mathrm{sgn}}$A near-ring (Wikipedia, nLab) "is" a ring in which addition is not necessarily commutative, and in which only half of the distributivity conditions hold (e.g. for "left near-rings" we can have $a(b+c)=ab+ac$, though not necessarily $(a+b)c=ac+bc$). Similarly, a near-semiring "is" a near-ring without additive inverses.
Indeed, the geometric realisation $$\displaystyle\coprod_{n=0}^{\infty}\mathrm{B}\mathrm{B}_n\simeq\coprod_{n=0}^{\infty}\mathrm{UConf}_{n}(\mathbb{R}^2),$$ of the braid category $\mathbb{B}$ seems to be a natural example of something we could call an "$(\mathbb{E}_2,\mathbb{E}_2)$$(\mathbb{E}_2,\mathbb{E}_1)$-near-semiring space" (perhaps the initial one), where:
The additive structure is induced by $(n,m)\mapsto n+m$ and by the group morphisms $$\oplus\colon\mathrm{B}_n\times\mathrm{B}_m\to\mathrm{B}_{n+m}$$ given by juxtaposition of braids:
The additive structure is induced by $(n,m)\mapsto n+m$ and by the group morphisms
$$\oplus\colon\mathrm{B}_n\times\mathrm{B}_m\to\mathrm{B}_{n+m}$$ given by juxtaposition of braids:

The multiplicative structure is induced by $(n,m)\mapsto nm$ and by the group morphisms $$\otimes\colon\mathrm{B}_n\times\mathrm{B}_m\to\mathrm{B}_{nm}$$ given by sending $(\sigma,\tau)$ to the braid obtained as follows:
The multiplicative structure is induced by $(n,m)\mapsto nm$ and by the group morphisms $$\otimes\colon\mathrm{B}_n\times\mathrm{B}_m\to\mathrm{B}_{nm}$$ given by sending $(\sigma,\tau)$ to the braid obtained as follows:- Partition the $nm$ strands into $n$ subsets of $m$ strands;
- Braid each of the $m$ strands according to $\tau$;
- Braid the $n$ subsets according to $\sigma$;
For example, the product of the braids $\sigma$ and $\tau$ depicted as
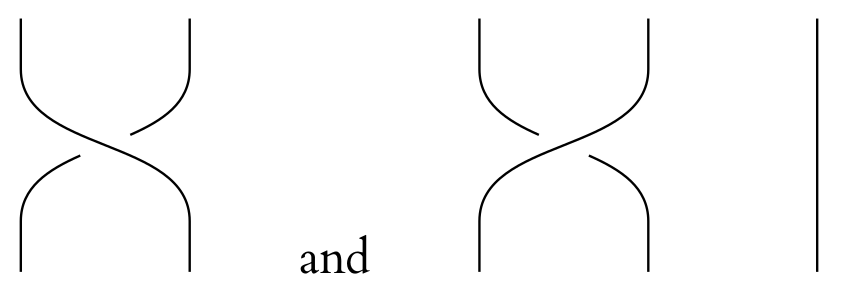
is given by
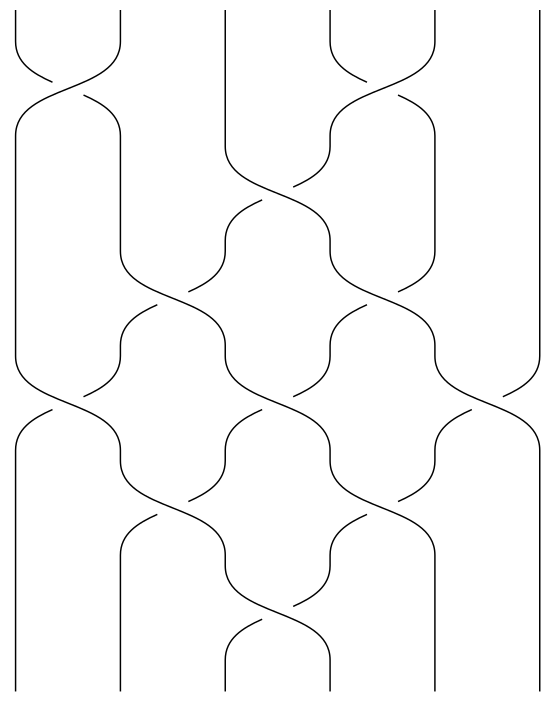
while $\tau\otimes\sigma$ is the braid
For example, the product of the braids $\sigma$ and $\tau$ depicted as
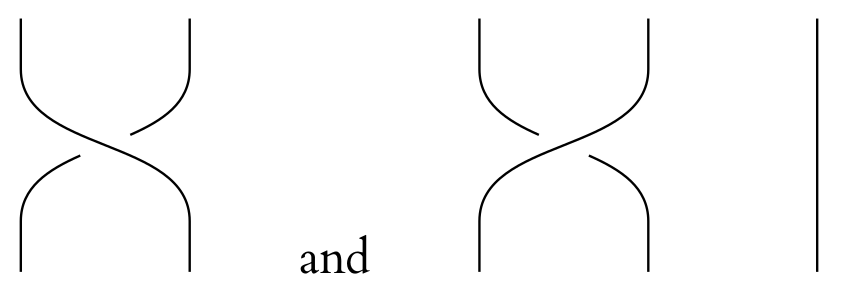
is given by

while $\tau\otimes\sigma$ is the braid
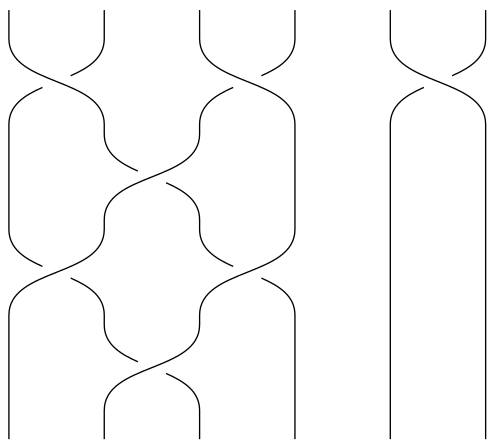
- The additive braiding
$$\beta^{\mathbb{B},\oplus}_{n,m}\colon n\oplus m\to m\oplus n$$ is given by the morphism of $\mathrm{Hom}_{\mathbb{B}}(n+m,n+m)\cong\mathrm{B}_{n+m}$ corresponding to the braid
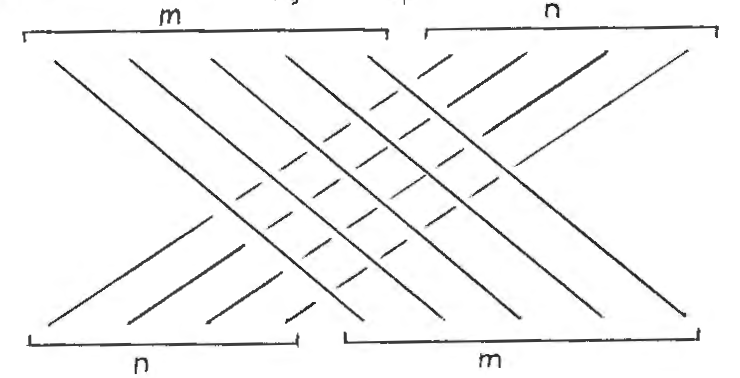
This is indeed a braiding:
- Naturality is given by the equality
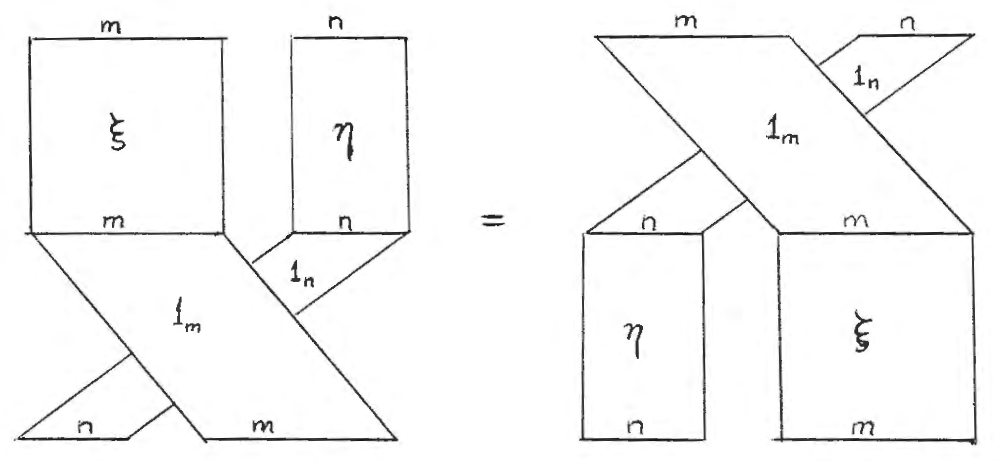
- The left hexagon equality is the equality of braids
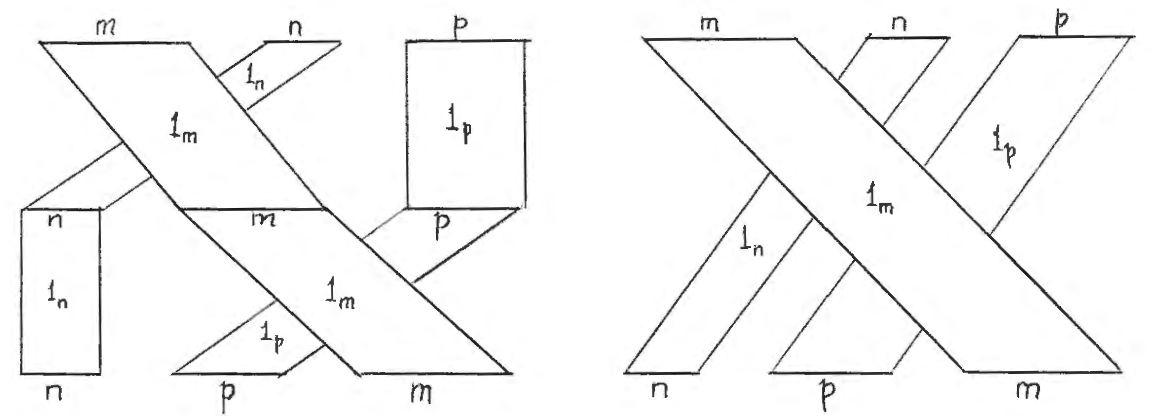
- The right hexagon equality is the same equality as above,but flipped:

The additive and multiplicative structures above are in a sense a lift of the ones in the groupoid of finite sets and permutations $$\coprod_{n=0}^\infty\mathrm{B}\Sigma_n\simeq\coprod_{n=0}^\infty\mathrm{UConf}_n(\mathbb{R}^\infty)$$ along the maps $\mathrm{B}_n\to\Sigma_n$ sending a braid to its underlying permutation.
They also relate to the additive structure of $\Pi_{\leq1}(\Omega^2S^2)$ via the abelianisation map $\deg\colon\mathrm{B}_n\to\mathbb{Z}$, satisfying the relations \begin{align*} \deg(\sigma\oplus\tau) &= \deg(\sigma)+\deg(\tau),\\ \deg(\sigma\otimes\tau) &= m^2\deg(\sigma)+n\deg(\tau). \end{align*}
(An interesting aside is that the compositions $$ \begin{array}{c} \mathbb{B} & \ra{} & \Pi_{\leq1}(\Omega^2S^2)\\ \da{}& & \da{}\\ \mathbb{F} & \ra{} & \Pi_{\leq1}(\Omega^\infty\mathbb{S}), \end{array} \qquad \begin{array}{c} \mathrm{B}_{nm} & \ra{\deg} & \mathbb{Z}\\ \da{}& & \da{\mathrm{mod}\text{ }2}\\ \Sigma_{nm} & \ra{\mathrm{sgn}} & \mathbb{Z}/2\\ \end{array} $$ play well with multiplication of braids in that we have \begin{align*} \deg(\sigma\otimes\tau) &= m^2\deg(\sigma)+n\deg(\tau)\\ &\equiv m\deg(\sigma)+n\deg(\tau)\ \text{(mod 2})\\ &\equiv m\sgn(\sigma)+n\sgn(\tau)\ \text{(mod 2})\\ &\equiv \sgn(\sigma\otimes\tau) \ \text{(mod 2}), \end{align*} where we used Fermat's little theorem for $p=2$.)
