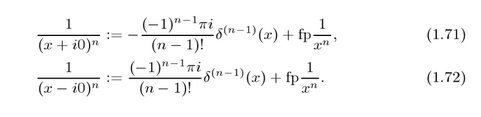According to Wolfram Alpha, it is $\frac{1}{\Gamma(-1/2)x^{3/2}}$. This, of course, involves regularization, so to get the full answer, we have to subtract the divergent part so that its Laplace transform to converge.
So, the answer is $ \frac{\sqrt{i}}2\delta ^{(1/2)}(x)+\text{f.p.}\frac{1}{\Gamma(-1/2)x^{3/2}}$, following Urs Graf's "Introduction to Hyperfunctions and Their Integral Transforms", page 36:
More explanation, using the theory of divergent integrals.
The Fourier transform of $x^n$ is $2\pi i^{n}\delta ^{(n)}(s )$. This means that at $s=0$ we have
$\int_{-\infty}^\infty x^ndx=2\pi i^n\delta ^{(n)}(0 )$.
If the integral is taken from zero, we have half of that value:
$\int_{0}^\infty x^ndx=\pi i^n\delta ^{(n)}(0 )$.
But there is an equality of divergent integrals
$\int_0^\infty \frac1{x^n} dx=\frac1{(n-1)!}\int_0^\infty x^{n-2} dx$
it is based on the fact that the following Laplace-based transform preserves the area under the integral, defining the equivalence class of divergent integrals:
$\int_0^\infty f(x)dx=\int_0^\infty\mathcal{L}_t[t f(t)]\left(x\right)dx=\int_0^\infty\frac1x\mathcal{L}^{-1}_t[ f(t)]\left(x\right)dx$
So, we have:
$\int_0^\infty \frac1{x^{n+1}} dx=\frac1{n!}\int_0^\infty x^{n-1} dx=\frac\pi{n!}i^{n-1}\delta ^{(n-1)}(0)$
For $n=3/2$, and adding coefficient $\frac{3/2}{\Gamma(-1/2)}$ to the both sides we get
$\frac{3/2}{\Gamma(-1/2)}\int_0^\infty \frac1{x^{5/2}} dx=\frac1{\Gamma(-1/2)\Gamma(1/2)}\int_0^\infty \sqrt{x} dx=\frac{\sqrt{i}\delta ^{(1/2)}(0)}{\Gamma(-1/2)\Gamma(1/2)}=-\frac{\sqrt{i}}2\delta ^{(1/2)}(x)$$\frac{3/2}{\Gamma(-1/2)}\int_0^\infty \frac1{x^{5/2}} dx=\frac1{\Gamma(-1/2)\Gamma(1/2)}\int_0^\infty \sqrt{x} dx=\frac{\sqrt{i}\delta ^{(1/2)}(0)}{\Gamma(-1/2)\Gamma(1/2)}=-\frac{\sqrt{i}}2\delta ^{(1/2)}(0)$
So to normalize our equation we should take this term with an opposite sign.