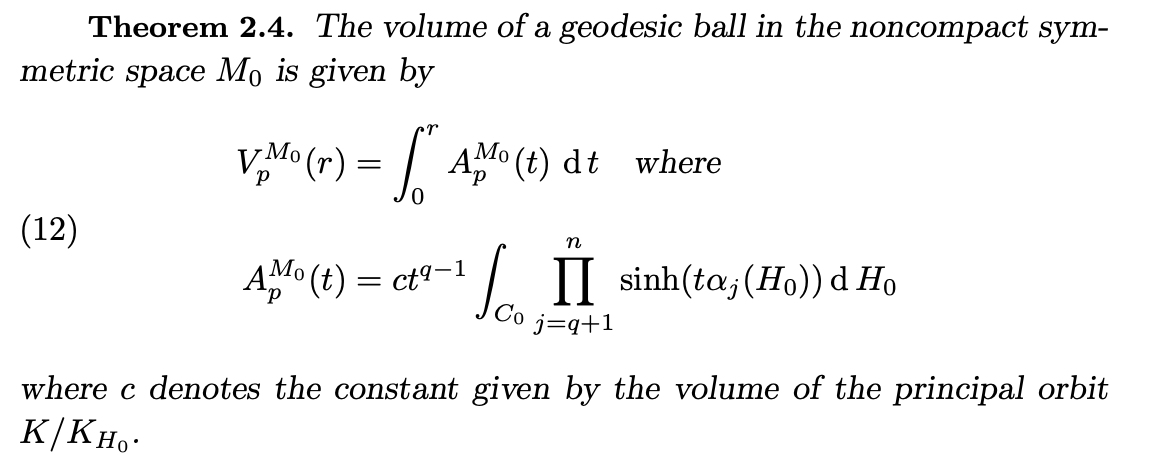$\DeclareMathOperator\SL{SL}\DeclareMathOperator\SO{SO}$Crossposted on MSE: https://math.stackexchange.com/questions/4261809/volume-of-a-geodesic-ball-in-sln-son
Question: What is the volume of a geodesic ball of radius $r$ in the symmetric space $\SL(n) / \SO(n)$$\SL(n) / {\SO(n)}$?
Context: Let $M = \SL(n) / \SO(n)$$M = \SL(n) / {\SO(n)}$. We can view $M$ as the Riemannian manifold of real $n\times n$ positive definite matrices with determinant 1 endowed with the so-called affine-invariant metric: $$g_P(U, V) = \mathrm{trace}(P^{-1} U P^{-1} V)$$$$g_P(U, V) = \operatorname{trace}(P^{-1} U P^{-1} V)$$ for $P \in M$ and $U, V \in \mathrm{Sym}(n)$$U, V \in \operatorname{Sym}(n)$ (symmetric $n \times n$ matrices).
Attempts/what I know:
(1) Theorem 2.4 of Theorem 2.4Gual-Arnau and Naveira - Volume of this papertubes in noncompact symmetric spaces (screenshot attached) provides a formula for the volume of a geodesic ball for any noncompact symmetric space. However, I am not very familiar with symmetric space theory, so I am unable to parse what this formula says for $\SL(n) / \SO(n)$$\SL(n) / {\SO(n)}$. Example 3.4.6 of the lecture notes Example 3.4.6 of these lecture notesSymmetric spaces (Frühlingssemester 2018) give a formula for the roots $\alpha_j$ used in Theorem 2.4, and it is well-known the rank is $q = n-1$. However, I do not understand how to determine the constant $c$. I have an idea what the domain $C_0$ might be (the intersection of a sphere and a Weyl chamber--expressionschamber—expressions for the Weyl chambers can be found), but I am not confident if this is correct.
(2) When $n=2$, we know $\SL(n) / \SO(n)$$\SL(n) / {\SO(n)}$ is isometric to the hyperbolic plane of curvature $-1/2$. However, I am looking for a formula for general $n$.
(3) It is well-known that $\SL(n) / \SO(n)$$\SL(n) / {\SO(n)}$ contains a totally geodesic submanifold isometric to a hyperbolic space. This gives us a lower bound on the volume of a ball. However, this is not sufficient for my purposes.
(4) $\SL(n) / \SO(n)$$\SL(n) / {\SO(n)}$ has constant Ricci curvature, so we can use the Bishop-GromovBishop–Gromov inequality to get an upper bound on the volume. However, this is not sufficient for my purposes.