For the uniform sampling from general convex polytopes, see e.g. Answer 1, Answer 2, other answers to this Question, and further references given there. Also, for instance, Fast MCMC Sampling Algorithms on Polytopes.
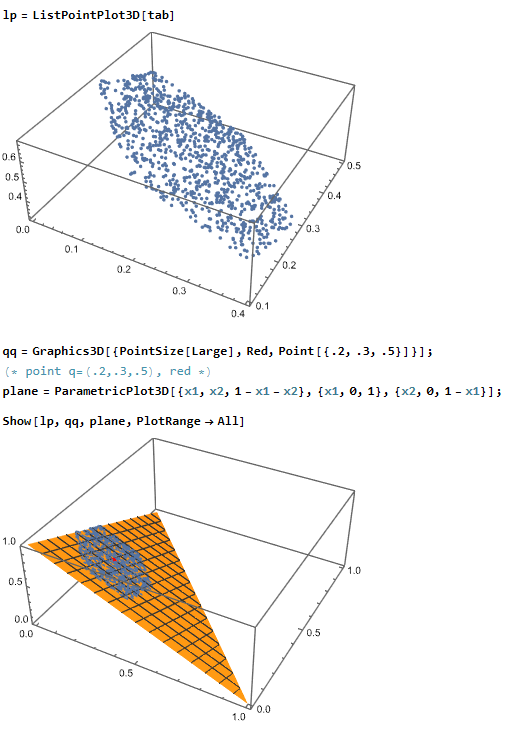
Here is an example with $1000$ sample points for $n=3$, $q=(.2, .3, .5)$, and $\epsilon=.4$, generated by a Markov Chain MonterMonte Carlo method (with $5$ steps for each of the $1000$ sample points: