The sum is equal to $$ \int\limits_{0}^{\infty}\frac{\exp(-x)}{1+W_0(x)}\,\mathrm{d}x = 0.7041699604... $$ where $W_0(x)$ is the Lambert-$W$ function.
Reference
Stephen Finch. "Errata and Addenda to Mathematical Constants [Math.HO]", §6.11. Iterated Exponential Constants, pg. 66.
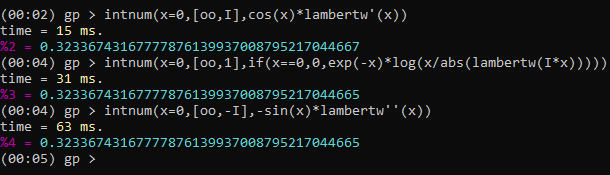
Two interesting divergent sums from this reference are as well $$\sum_{n=1}^\infty (-1)^{n-1} (2n)^{2n-1} = \int\limits_{0}^{\infty}e^{-x}\log(x/|W_{0}(i x)|)\mathrm{d}x = 0.3233674316...$$ which seems to be also the cosine and sine integrals of the derivatives $W'_{0}(x) = \frac {W_{0}(x)}{x(1 + W_{0}(x))}$ and $-W''_{0}(x)$ respectively $$\sum_{n=1}^\infty (-1)^{n-1} (2n)^{2n-1} = \int\limits_{0}^{\infty}\mathrm{cos}(x) W'_{0}(x)\mathrm{d}x = -\int\limits_{0}^{\infty}\mathrm{sin}(x) W''_{0}(x)\mathrm{d}x$$ as it can be seen in the following Pari GP v2.14.0 lines
although, as it is pointed out, a rigorous proof is not yet known.
And this variation, $$\sum_{n=1}^\infty (-1)^{n-1} (2n-1)^{2n} = \frac{1}{2i}\int\limits_{0}^{\infty}e^{-x}(g(ix)-g(-ix))\mathrm{d}x = 0.0111203007...$$ where $g(x) = W_{0}(x)/(1 + W_{0}(x))^3$