$\newcommand{\R}{\mathbb R}$Let $p:=\rho$, $H:=H[p]$, $G:=G[p]$. Let us show that \begin{equation*} f(x)\equiv kx \end{equation*} with \begin{equation*} k=1/14334 \end{equation*} will do. We will not need the restriction that the support of the distribution is $[0,\infty)$.
By approximation, without loss of generality (wlog), $p(x)>0$ for all real $x$ -- for instance, one may approximate $p$ by its convolution $p*g$ with the density $g$ of a centered normal distribution with an arbitrarily small variance. Then $p*g>0$ on $\R$ and $p*g$ is arbitrarily close to $p$ and log concave, since $p$ and $g$ are log concave -- see e.g. this Wikipedia article.
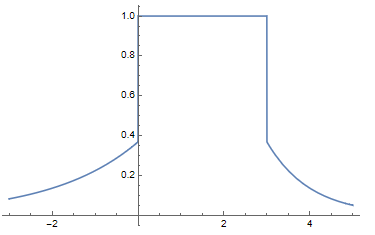
Therefore and because $p$ is a log-concave density, $p$ is continuous and attains its maximum value, say $p_*[>0]$, at some point $c\in\R$, so that $p_*=p(c)\ge p(x)$ for all real $x$. Moreover, again because $p$ is a log-concave density, there exist (unique) real $a$ and $b$ such that \begin{equation*} \text{$a<c<b$ and $p(a)=p(b)=p_*/e$. } \end{equation*} Again by the log-concavity of $p$, \begin{equation*} p(x)\le q(x):= \left\{ \begin{aligned} q_1(x):=p_*\exp\Big\{-\frac{x-c}{a-c}\Big\} &\text{ if }x<a, \\ p_* &\text{ if }a\le x<b, \\ q_2(x):=p_*\exp\Big\{-\frac{x-c}{b-c}\Big\} &\text{ if }x\ge b. \end{aligned} \right. \end{equation*} As an illustration, for $p(x)=xe^{-x}1(x>0)$, here are the graphs $\{(x,p(x))\colon-2\le x\le6\}$ (blue), $\{(x,q(x))\colon-2\le x\le6\}$ (black), $\{(x,q_1(x))\colon a\le x\le c\}$ (dashed), and $\{(x,q_2(x))\colon c\le x\le b\}$ (dashed):
For this particular $p$, we have $c=1$, $a=-W_0\left(-1/e^2\right)=0.15859\dots$, and $b=-W_{-1}\left(-1/e^2\right)=3.1461\dots$, where $W_j$ is the $j$th branch of the Lambert $W$ function.
By shifting, wlog $$a=0.$$ So, \begin{align*} G&\le\iint\limits_{\R^2} q(x)q(y)|x-y|\,dx\,dy \\ &=p_*^2\frac{e^2 b^3+9 e b^3+3 b^3+3 b^2 c-12 e b^2 c-3 b c^2+12 e b c^2}{3 e^2} \\ &\le p_*^2\frac{\left(1+3 e+e^2/3\right) b^3}{e^2}, \end{align*} since $0<c<b$. Moreover, again by the log-concavity of $p$, we have $p\ge p_*/e$ on the interval $[a,b]=[0,b]$, so that $1=\int_\R p\ge\int_0^b p_*/e=bp_*/e$, whence $p_*\le e/b$ and \begin{equation*} G\le (1+3 e+e^2/3) b. \tag{1} \end{equation*}
On the other hand, because $p\ge p_*/e$ on the interval $[a,b]=[0,b]$ and the integrand in the definition of $H$ is $\ge0$, we have \begin{align*} H&\ge\Big(\frac{p_*}e\Big)^3\iiint\limits_{[0,b]^3} \Big(\frac{|x-z|+|y-z|}2 -\Big|\frac{x-z+y-z}2\Big|\Big)\,dx\,dy\,dz \\ &=\Big(\frac{p_*}e\Big)^3\frac{b^4}{24}. \end{align*} Also, $1=\int_\R p\le\int_\R q=p_*b(1+1/e)$, so that $p_*\ge1/(b(1+1/e))$ and hence \begin{equation*} H\ge\Big(\frac1{(e+1)b}\Big)^3\frac{b^4}{24}=\frac b{24(e+1)^3}. \tag{2} \end{equation*}
Comparing (1) and (2), we get \begin{equation*} H\ge\frac G{24(e+1)^3(1+3 e+e^2/3)}\ge\frac G{14334}, \end{equation*} as claimed.