Remark 1: To show without Mathematica that $\max(\de_1,\de_2)\ge\frac{24}{121}\,\ep$ in case (ii), one can do as follows. Rewrite the conditions $\de_1\ge m(a)\ep$ and $\de_2\ge-\de_1/n(a)+\frac{(3/4-a)^2}{(1-a)^2}\,\ep$ as
\begin{equation}
\de_1/\ep\ge\max(A,(B-\de_2/\ep)K),
\end{equation}
where $A:=m(a)$, $B:=\frac{(3/4-a)^2}{(1-a)^2}$, and $K:=n(a)$. If $\de_2\ge\frac{24}{121}\,\ep$, we are done. Otherwise,
\begin{equation}
\de_1/\ep\ge
\max(m(a),M(a)),
\end{equation}
where
\begin{equation}
M(a):=(B-\tfrac{24}{121})K=\Big(\frac{(3/4-a)^2}{(1-a)^2}-\frac{24}{121}\Big)n(a).
\end{equation}
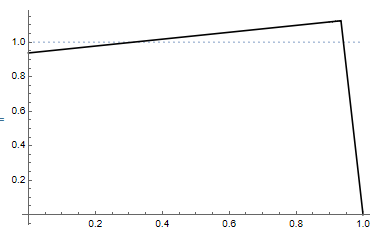
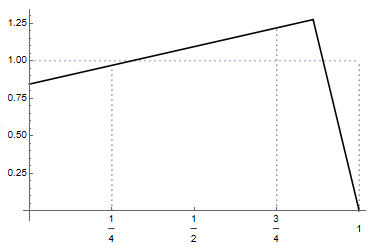
Note that $m$ is increasing on $[0,1]$, $M$ is decreasing on $[1/4,1/2]$, and $m(\frac 5{16})=\frac{24}{121}=M(\frac 5{16})$. It follows that for all $a\in[1/4,1/2]$
\begin{equation}
\de_1/\ep\ge\max(m(a),M(a))\ge\tfrac{24}{121},
\end{equation}
as was proved by Mathematica.
Remark 2: Following the lines of the consideration of case (ii) (with $a=5/16$, $\ep\downarrow0$, and $b\uparrow1$), one can see that the factor $\frac{24}{121}=0.19834\dots$ in the lower bound $\frac{24}{121}\,\ep$ in (*) is the best possible.