Let $z_1,z_2,\dots,z_n$ be $n$ complex numbers distributed uniformly and randomly over the unit disc $x^2+y^2 \leq 1$. Let $z$ be the complex number defined by the mean of the of these numbers,that is,
$$ z=\frac1n \sum_{i=1}^{n} z_i.$$
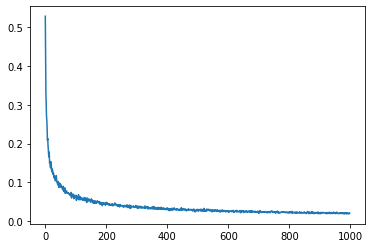
Numerical simulations reveal that $E(|z|)$ exists. I want to know if there is some analytical way of finding a closed form expression for the expected radial distance of $z$ in terms of $n$? Any hints/responses will be greatly appreciated . The graph shows the variation of $E(|z|)$ with $n$