Consider generalizing the last line to
Let $I$ be a point on $B'C'$, and let $FI$ meet $AB$, $AC$ at $H$, $G$.
Then the cross ratio of $H,F,G,I$ is the negative golden ratio: $$\frac{HG\cdot FI}{FG\cdot HI}=-\frac{1+\sqrt{5}}{2} = -\phi$$
The original version is the limit of the general version as $I$ goes to infinity.
But the general version is projectively invariant, so we can prove it using coordinates where $A$ is at vertical infinity, the conic is $y=x^2$, and the tangent at $A$ is the line at infinity.
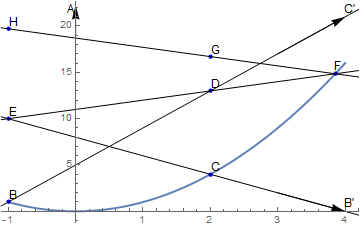
Then the first coordinates are simple: \begin{align} A &= \infty(0,1)\\ B &= (b,\ b^2)\\ C &= (c,\ c^2)\\ A' &= ((b+c)/2,\ bc)\\ B' &= \infty(1,2c)\\ C' &= \infty(1,2b)\\ D &= (c,\ (b-c)^2+c^2)\\ E &= (b,\ (b-c)^2+b^2)\\ F &= ((1-\phi)b+\phi c,\ ((1-\phi)b+\phi c)^2)\\ \end{align} and we can stop computing coordinates there. The graphic below shows the case $b=-1$, $c=2$.
(There is also another solution for $F$, not mentioned in the original question, which leads to switching $\phi$ and $1-\phi$ in the above and the below.)
We want to evaluate the cross ratio $(HG\cdot FI)/(FG \cdot HI)$. Since $I$ is a point at infinity, the $FI$ and $HI$ terms will cancel. Then we can compute the directed quotient $HG/FG$ from the $x-$coordinates, which are $c$ and $b$ for $G$ and $H$. This gives us the desired result: $$\frac{HG\cdot FI}{FG \cdot HI}=\frac{HG}{FG}=\frac{b-c}{(b-c)(1-\phi)}=-\phi$$