Return to Answer

The $C_6$ case

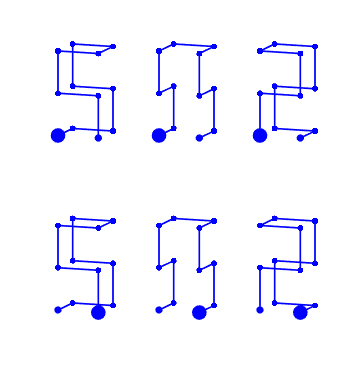
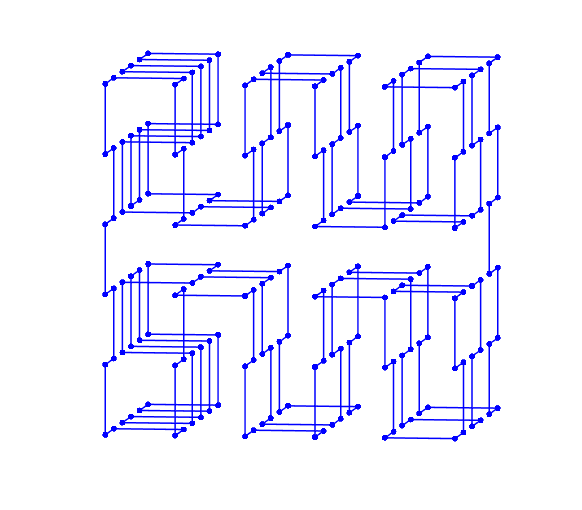
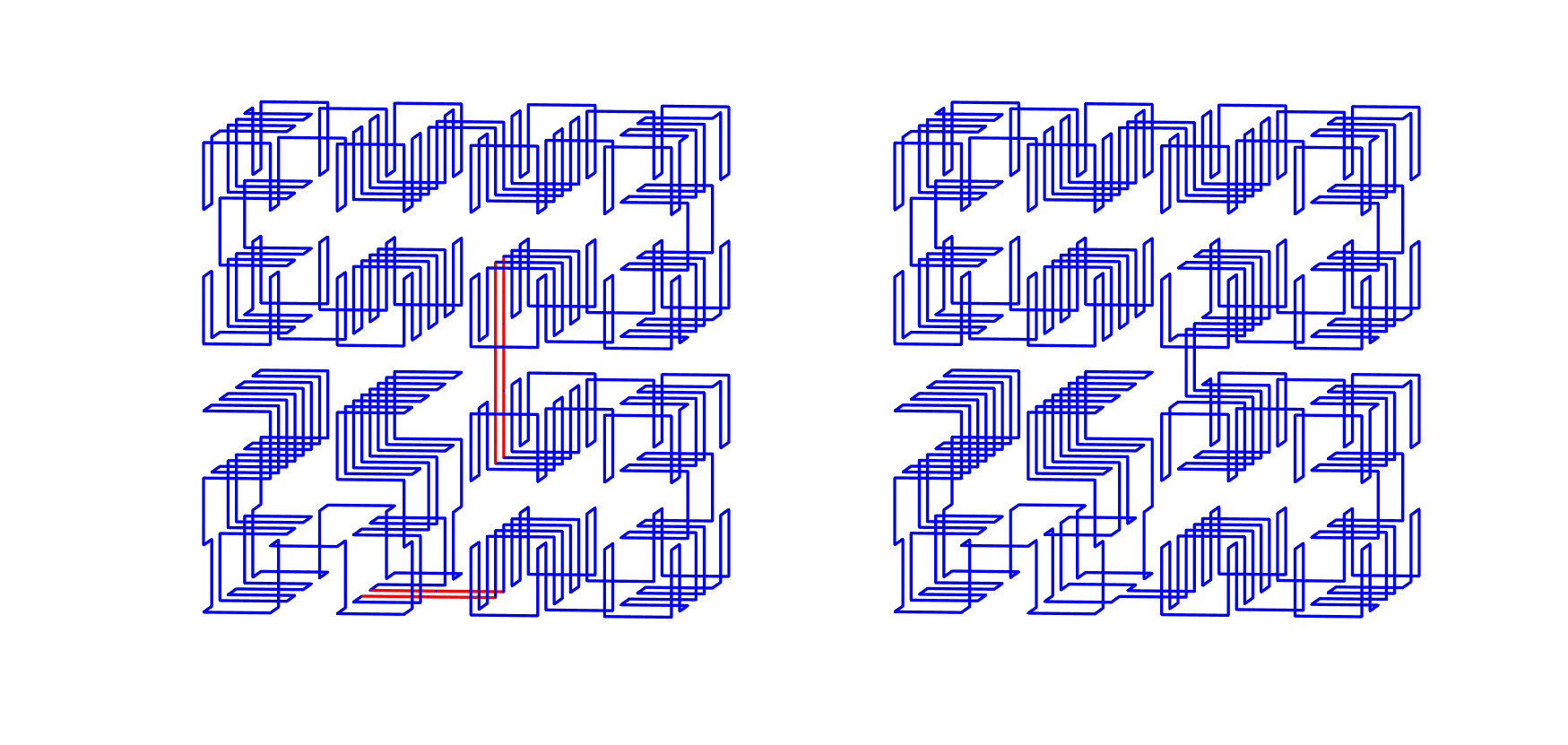
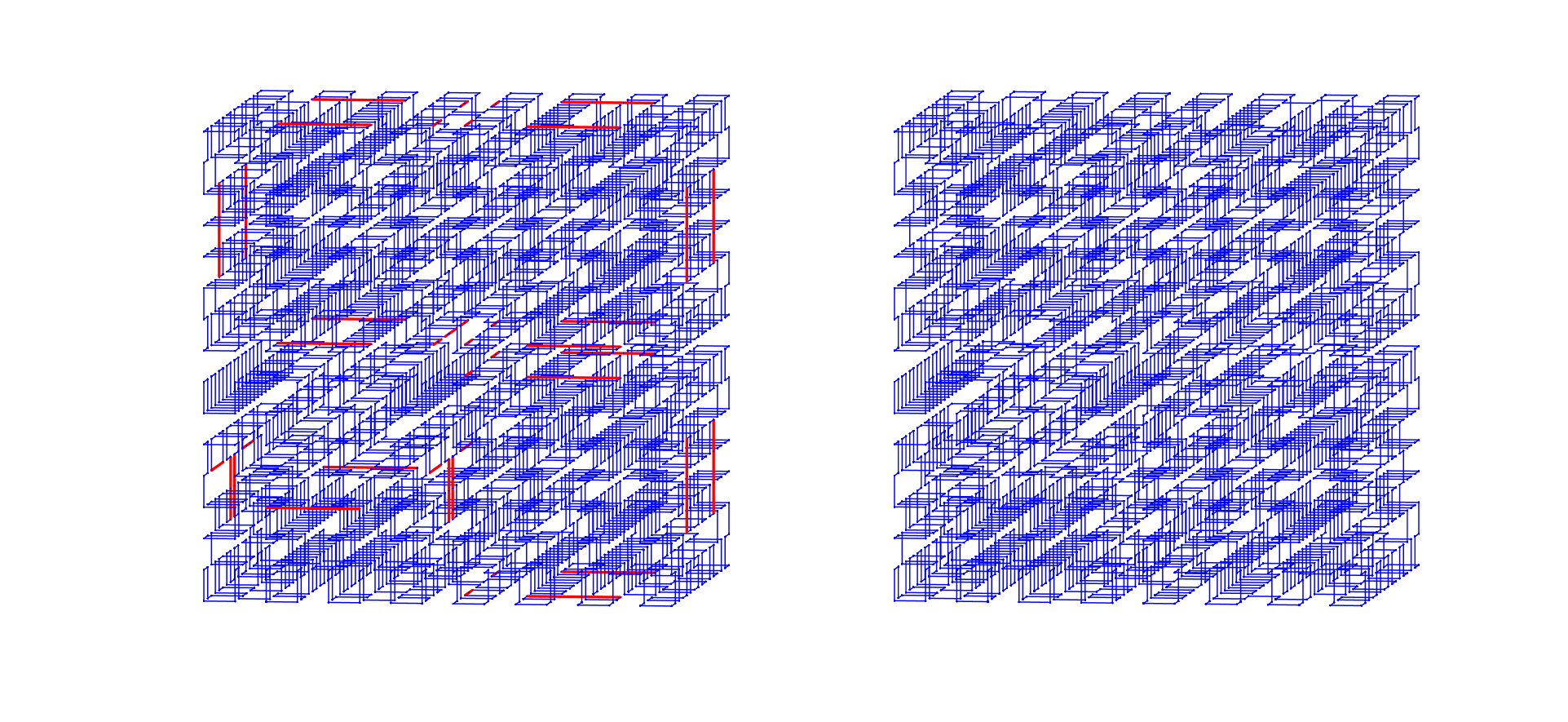
Update: $C_6$ is also possible. We use the following six primitives, each implementing a Hamiltonian path in a $(2 \times 2 \times 3)$ box (big dot indicates entrance). Arranging nine of them suitably, we can fill a $(6 \times 6 \times 3)$ box; this is the top half of our solution. The bottom half is its mirror image.

1. Top left: Both green edges are collinear with the adjoining blue edges. Both defects are fixed by mirroring our curve (top right).
2. Middle left: Only one of the green edges is collinear with its blue edge. The other green is collinear with the rod. Again we mirror the curve (middle right).
3. Bottom left: Only one of the green edges is collinear with its blue edge. The other green is orthogonal to its blue and to the rod. This is problematic: mirroring does not help. Here we replace our curve with a different Hilbert curve (bottom right).

1. Top left: Both green edges are collinear with the adjoining blue edges. Both defects are fixed by mirroring our curve (top right).
2. Middle left: Only one of the green edges is collinear with its blue edge. The other green is collinear with the rod. Again we mirror the curve (middle right).
3. Bottom left: Only one of the green edges is collinear with its blue edge. The other green is orthogonal to its blue and to the rod. This is problematic: mirroring does not help. Here we replace our curve with a different Hamiltonian path on the $C_2$ (bottom right).