I'll prove that for any triangle below, $\displaystyle \theta \geqq \pi /3\ $
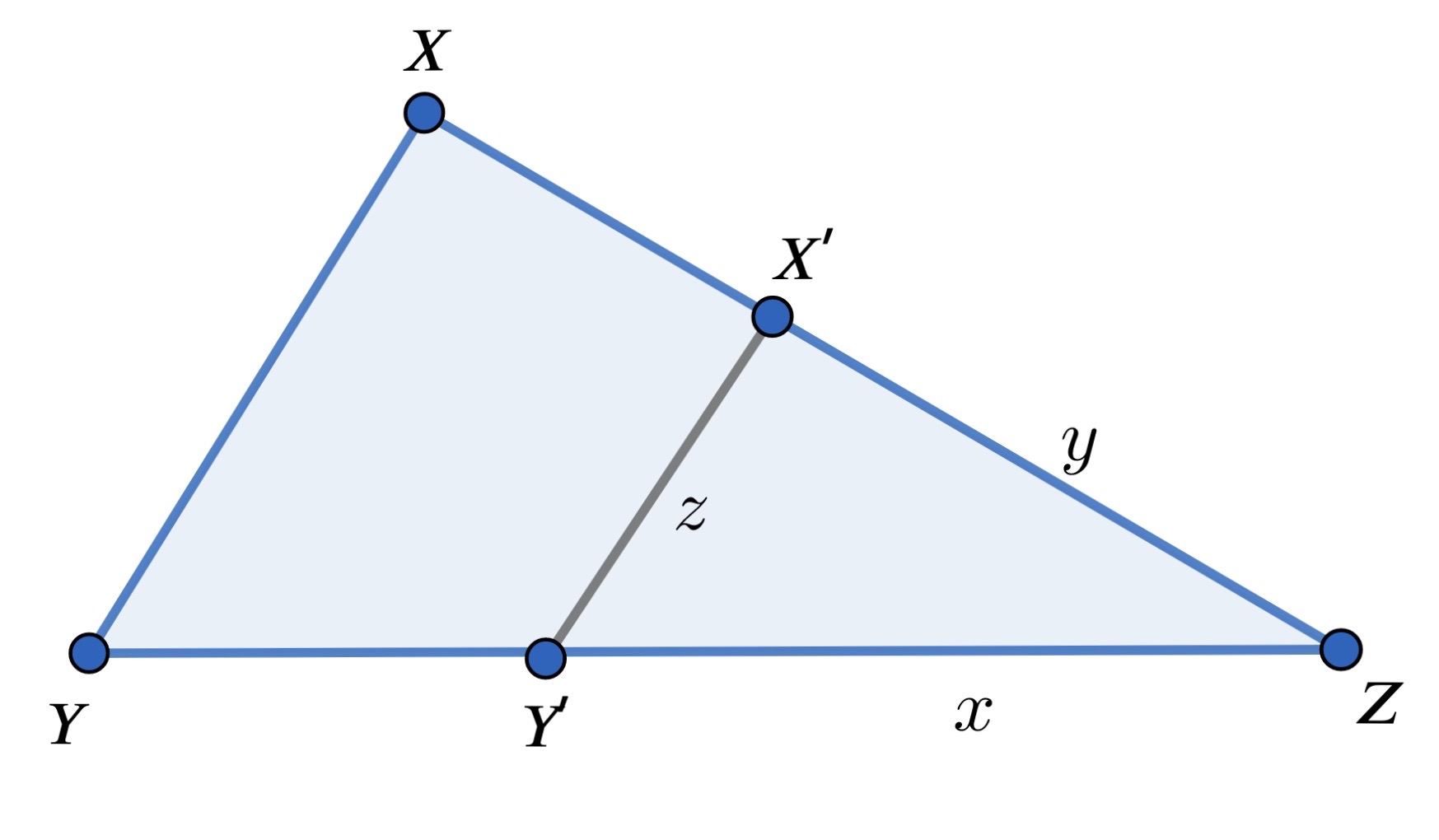
Assuming $\displaystyle X'Y' =z$ be the line segment that bisects area $\displaystyle A$ of triangle $\displaystyle XYZ$ .
Let $\displaystyle \angle Z=\alpha $ , $\displaystyle Y'Z=x$ and $\displaystyle X'Z=y$
By Heron's formula, $\displaystyle \frac{A}{2} =\frac{1}{4}\sqrt{( x+y+z)( x+y-z)( x-y+z)( -x+y+z)} =\frac{1}{4}\sqrt{( 2xy)^{2} -\left( x^{2} +y^{2} -z^{2}\right)^{2}}$ ,
so we get $\displaystyle z=\sqrt{x^{2} +y^{2} -2\sqrt{( xy)^{2} -A^{2}}} \geqq \sqrt{2xy-2\sqrt{( xy)^{2} -A^{2}}}$ .
On the other hand, $\displaystyle \frac{A}{2} =\frac{1}{2} xy\sin \alpha $, so $\displaystyle xy=\frac{A}{\sin \alpha }$,
and we get $ $$\displaystyle z\geqq \sqrt{2A\left(\frac{1}{\sin \alpha } -\frac{1}{\tan \alpha }\right)} =\sqrt{2A\tan\frac{\alpha }{2}}$
When $\displaystyle x=y$ and $\displaystyle \alpha $ be the smallest angle of triangle $\displaystyle XYZ$, $\displaystyle z$ get the minimum value.
and then $\displaystyle \theta =\frac{\pi -\alpha }{2}$, so $\displaystyle \theta \geqq \pi /3\ $.