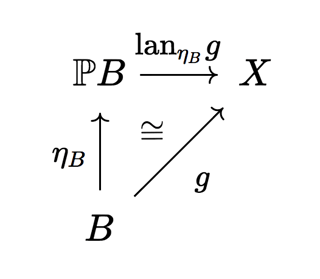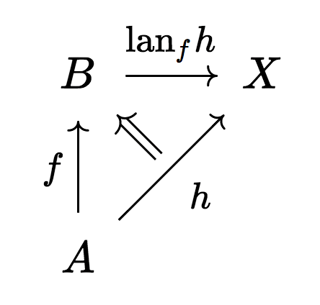This is a question for which the theory of (co)KZ doctrines is well-suited, in which (co)completeness properties are characterised by the existence of certain extensions. I'll choose to work with KZ doctrines, and thus cocompleteness properties. A good reference is Walker's Distributive laws via admissibility, and the full definitions of the various concepts I make use of below can be found there.
Let $(\mathbb P, \eta)$ be a KZ doctrine on a 2-category $\mathcal K$. An object $X \in \mathcal K$ is $\mathbb P$-cocomplete if for all $g \colon B \to X$, there exists a left extension $\mathrm{lan}_{\eta_B} g$, exhibited by an invertible 2-cell,
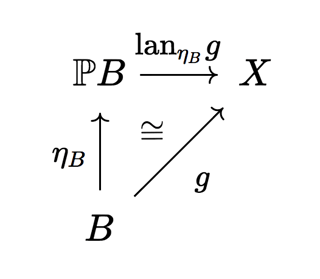
such that the left extension respects those defined by the KZ doctrine in an appropriate sense (q.uiver link).
From Marmolejo–Wood's Kan extensions and lax idempotent pseudomonads, we know that the $\mathbb P$-cocomplete objects are equivalently the pseudoalgebras for the pseudomonad $\mathbf P \colon \mathcal K \to \mathcal K$ induced by $(\mathbb P, \eta)$, so that when we take $\mathbf P$ to be a pseudomonad for a cocompletion under a class of weights $\Phi$, then $\mathbb P$-cocompleteness corresponds to admitting all $\Phi$-colimits. In particular, for $\mathbb P$ the small presheaf construction on locally small categories, a $\mathbb P$-cocomplete object is just a small-cocomplete locally small category.
To relate this to your question, we also need to introduce the notion of admissibility for a KZ doctrine. A 1-cell $f \colon A \to B$ is $\mathbb P$-admissible if, for any $h \colon A \to X$ for $X$ a $\mathbb P$-cocomplete object, there exists a left extension $\mathrm{lan}_f h$,
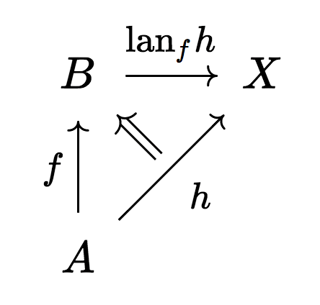
such that the left extension is preserved by $\mathbb P$-cocontinuous 1-cells into any $\mathbb P$-cocomplete object (q.uiver link).
Crucially, as Walker observes in Remark 25 ibid., a $\mathbb P$-admissible 1-cell is $\mathbb P$-fully faithful (meaning $\mathbf Pf$ is representably fully faithful) if and only if every left extension as in the diagram above is exhibited by an invertible 2-cell.
Taking $\mathbb P$ to be the small presheaf construction, the $\mathbb P$-admissible 1-cells are the functors $f \colon A \to B$ for which $B(f{-}, b)$ is a small presheaf for all $b \in B$. We shall call these small functors. (Ivan Di Liberti mentions several equivalent conditions for a functor to be small in this answer.) The $\mathbb P$-fully faithful 1-cells are precisely the fully faithful functors. So, if a locally small category $X$ is small-cocomplete, every functor $A \to X$ admits a left extension along small functors $A \to B$ (hence $X$ is "lax-injective" with respect to small functors). Furthermore, these left extensions are exhibited by invertible 2-cells precisely for those small functors $A \to B$ that are fully faithful (hence $X$ is "pseudo-injective" with respect to small fully faithful functors).
Conversely, if a locally small category $X$ admits left extensions of functors $A \to X$ along small functors, it in particular admits a left extension along the Yoneda embedding of $A$ (since the unit $\eta$ of a KZ doctrine is $\mathbb P$-admissible), and hence is small-cocomplete. Therefore, the locally small categories that are lax-injective with respect to small functors are precisely the small-cocomplete categories.
One question remains, which regards the case when $X$ is only known to admits left extensions along $\mathbb P$-fully faithful $\mathbb P$-admissible 1-cells. Here, we may restrict our consideration to the fully faithful KZ doctrines, which are those for which the components of the unit $\eta_B \colon B \to \mathbb P B$ are representably fully faithful. In this case, $X$ once again admits extensions along $\eta_B$, and hence is $\mathbb P$-cocomplete. Since the Yoneda embedding is fully faithful, the small presheaf construction is a fully faithful KZ doctrine. Therefore, the locally small categories that are pseudo-injective with respect to small fully faithful functors are precisely the small-cocomplete categories.
(Let me know if something doesn't quite look right – I could well have made a mistake somewhere along the line!)