Let $$h(u):=u^3 \Big|\int_u^{\infty } \frac{e^{-i t}}{t^3} \, dt\Big|$$$$h(u):=u^3 \left|\int_u^\infty \frac{e^{-i t}}{t^3} \, dt\right|$$ for $u>0$. Is the function $h$ concave on $(0,\infty)$?
(For context, see Proposition 4.4.4 and formula (4.4.21) in this paper or, equivalently, Proposition 4.4 and formula (4.21) in the corresponding arXiv preprint. In particular, according to that proposition, $h(v^{1/6})^2$ is concave in $v>0$.)
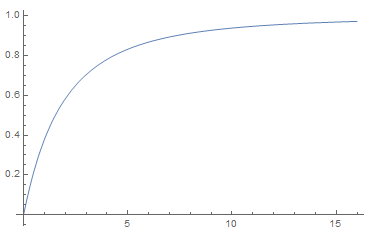
Here is the graph $\{(u,h(u))\colon0<u<16\}$$\{(u,h(u)):0<u<16\}$: