Can we prove, without using Laguerre polynomials, that $f_n(x)=O(\frac{n!}{\sqrt{n}})$ i.e. that
$$ \exists C>0, \exists N\in\mathbb N, \forall x\geq0, \forall n\geq N :\ \big| f_n(x) \big|\leq C \frac{n!}{\sqrt{n}}, $$
where $$ f_n(x)=e^{\frac{x}{2}}\int^\infty_0 e^{-t}t^{n-\frac{1}{2}}\cos(2\sqrt{xt})dt,\quad x \geq 0\;? $$
Proof (by using Laguerre polynomials): it's easy to show that
$$
f_n(x)=\sqrt \pi e^{-x/2} n! L^{(-1/2)}_n(x)
$$
and we know that
$$
L^{(-1/2)}_n(x)=O\Big(e^{x/2}\frac{1}{\sqrt{n}}\Big).
$$
My reference is page 9 formula 18 $$L^{\alpha }_n(x)=O\Big(e^{\frac x2}x^{\frac{-\alpha}2 -\frac 14}n^{\frac{\alpha}2 -\frac 14} \Big) .$$ or see The polynomials' asymptotic behaviour for large n 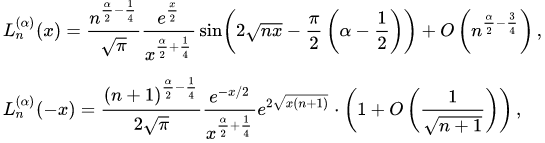
However, I'd like to not use this simple argument.
