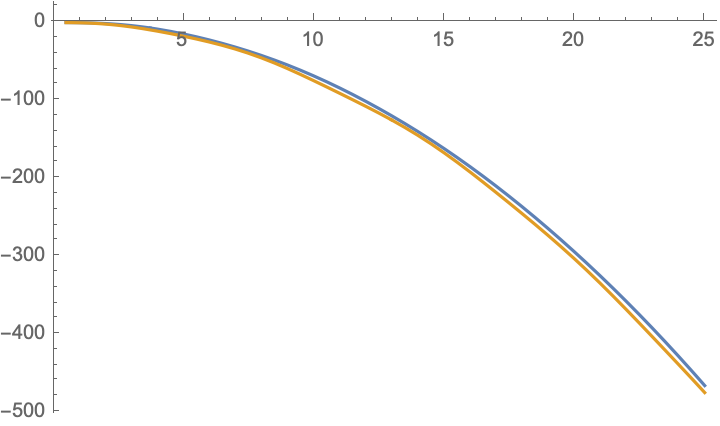
For large $n$ you may approximate the sum by an integral, which gives $$\sum_{k=1}^{n} k \sin (x/k)-nx\simeq \int_0^\infty\bigl(k\sin(x/k)-k\bigr)\,dk=-\tfrac{1}{4}\pi^2 x^2.$$$$\sum_{k=1}^{n} k \sin (x/k)-nx\simeq \int_0^\infty\bigl(k\sin(x/k)-k\bigr)\,dk=-\tfrac{1}{4}\pi x^2.$$ The plot compares the left-hand-side of this equation for $n=1000$ (blue curve) with the right-hand-side (gold) as a function of $x$.