A few more interesting plots, including a spectacular one that I call The Eye of The Zeta Function (the last one on this post).
The series for $\phi_1$ and $\phi_2$ (see Appendix in my original question) converge very slowly and in a chaotic way depending on $\sigma$ and $t$. A better solution is to use the Riemann-Siegel formula.
Slow, chaotic convergence of the series
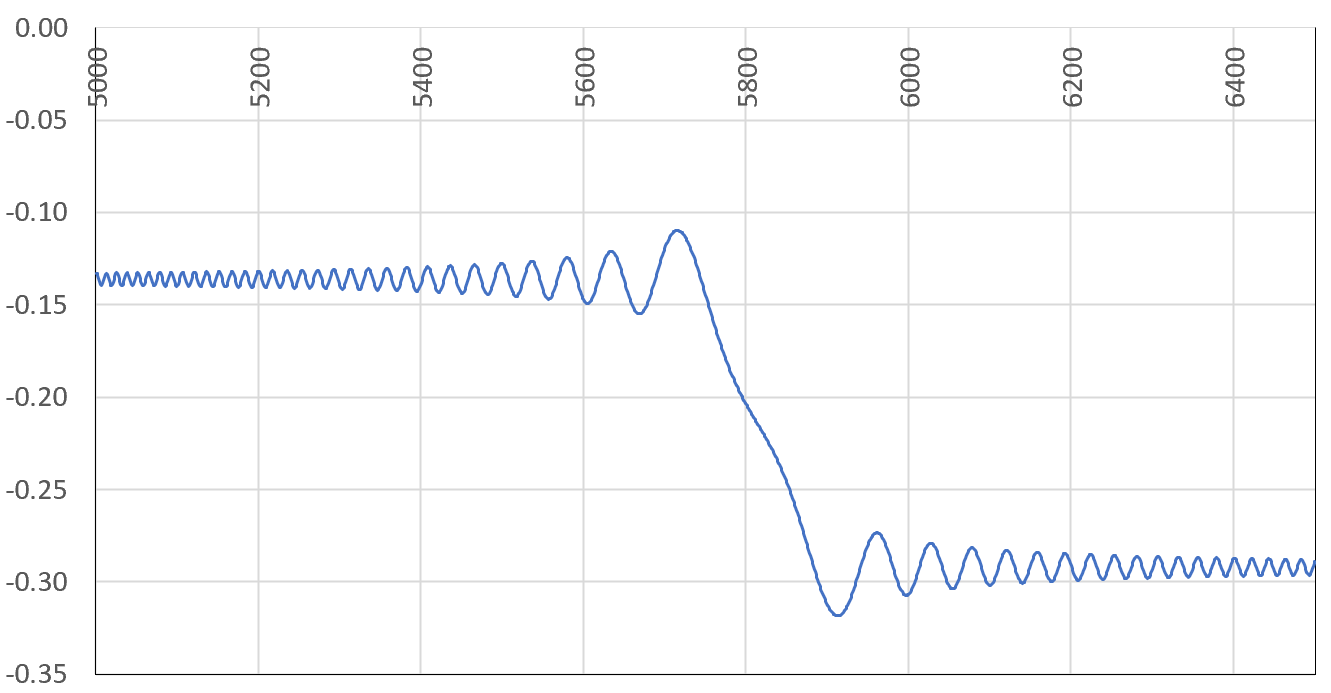
If for some large $n$ in the series, the quantity $t\log(n+1) - t\log n \approx t/n$ is close to an odd multiple of $\pi$, then around that $n$, a lot of terms in the series will have the same sign and similar value (as opposed to the regular alternating behavior), and if $n$ is not large enough, a sum that seems to have converged before $n$ will suddenly experience a huge shift. This is illustrated below.
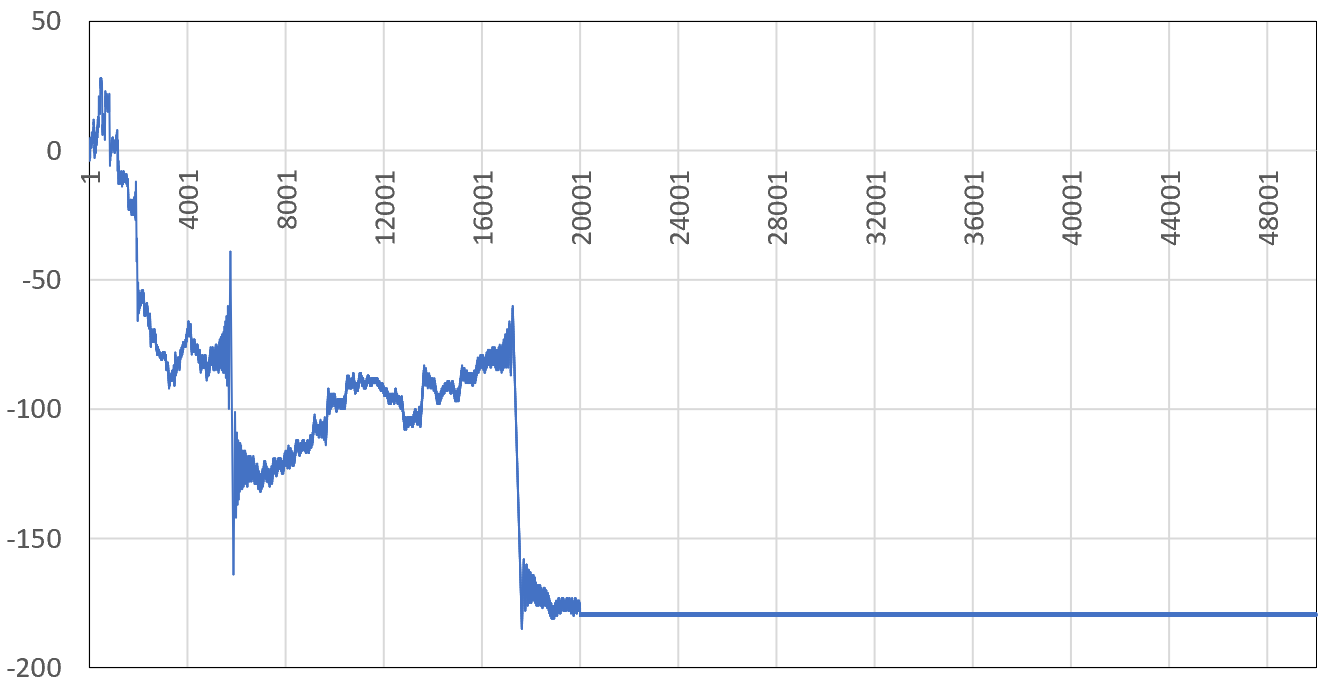
In the above figure, the sum for $\phi_2$ with $\sigma=0.75$ and $t=18265.2$ after seemingly converging to around $-0.135$ using the first 5000 terms, experiences a massive drop between $n=5760$ and $5860$ before stabilizing at around $-0.292$ (the correct value confirmed by Mathematica). The next chart shows the cumulative sum of the term signs, for consecutive terms of the series up to $n=50000$. A huge drop or increase means many successive terms having the same sign around the $n$ in question (the X-axis represents $n$), explaining the mechanism at play in the first chart.
Note that when $n$ is large enough, the series (at least in this example, around $n=20,000$) eventually becomes almost perfectly alternating: the blue curve in the previous plot becomes an almost flat horizontal line.
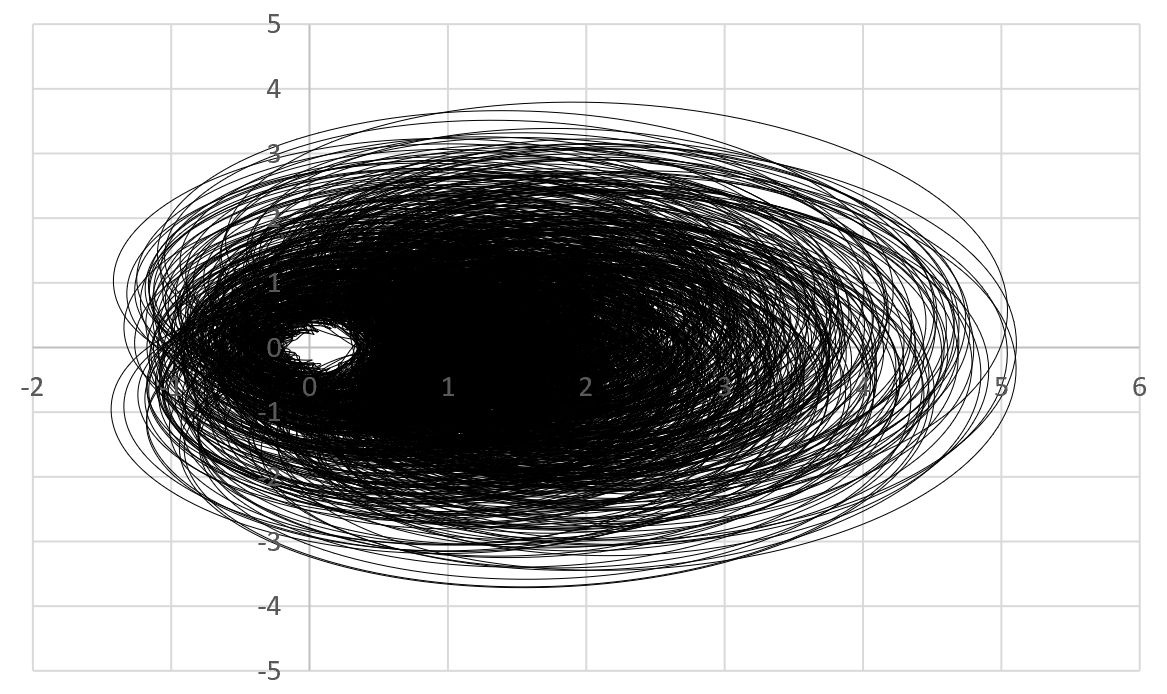
The eye of the Zeta function
Below is the scaled orbit of the Zeta function for $\sigma=0.75$ and $0<t<3000$, featuring $300,000$ points $(X(t),Y(t))$, with $X(t)=\phi_1(\sigma,t)$, $Y(t)=\phi_2(\sigma,t)$, and using increments of $0.01$ for $t$. What you see below is just one slice corresponding to $\sigma=0.75$. The whole orbit (if you allow $\sigma$ to vary in $]\frac{1}{2},1[$) would densely cover a 3-D volume in space.
There is a hole (the "eye") around $(0,0)$ suggesting that $\zeta(\sigma+it)$ has no root if $\sigma=0.75$. It looks like there is an absolute positive constant $\epsilon_\sigma$ (not depending on $t$) such that $X^2(t)+Y^2(t) > \epsilon_\sigma$$X^2(t)+Y^2(t) \geq \epsilon_\sigma$ for all $t$'s if $\sigma \neq \frac{1}{2}$. The million-dollar question is this: Do we have $\epsilon_\sigma \neq 0$? Of course, for the limiting case $\sigma=\frac{1}{2}$, we have $\epsilon_\sigma = 0$ since $\zeta$ has at least one root (indeed infinitely many) on the critical line.
Getting this plot right is not easy, since the series for $\phi_1,\phi_2$ converge in a chaotic way and require convergence boosting techniques (see here). Also using increments of $0.01$ is OK for small values of $t$, but as $t$ becomes larger, the oscillations in $\phi_1,\phi_2$ get exacerbated, requiring smaller and smaller increments to get a good coverage of the orbit, and to avoid missing a potential root.
My next step is to randomize $\phi_1,\phi_2$, possibly replacing $\cos(\gamma + t\log n)$ by $Z_n \cdot \cos(\gamma + t\log n)$, where $Z_1,Z_2\dots$ are i.i.d. Bernoulli-like random variables. I've already spent many hours on that, to no avail so far. Along similar lines, see this paper discussing the complete Riemann Zeta distribution.