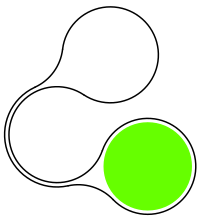
Ionin–Pestov theorem is not very well known, but it deserves to be included in standard introductory texts on differential geometry of curves. It gives the simplest meaningful example of a local-to-global theorem which is what differential geometry is about.
Theorem. Assume that a plane region $F$ is bounded by a simple loop with curvature at most $1$. Then $F$ contains a unit disc.
The original reference:
- Пестов, Г. Г., Ионин В. К. О наибольшем круге, вложенном в замкнутую кривую // Доклады АН СССР. — 1959. — Т. 127, № 6.
We used it in our textbook What is differential geometry.