This answer assumes that $G$ is finite. We can then assume that $G$ consists of orthogonal transformations (just use a $G$-invariant inner product).
Choose a point $x\in\Bbb Q^n$ that is not fixed by any non-identity $g\in G$. Consider the Voronoi decomposition of $\Bbb R^n$ w.r.t. the orbit $G\cdot x$. Some thought reveals that the Voronoi cells are cones with apex at the origin and spanned by rational vectors. Furthermore, $G$ acts regularly (that is, transitively and freely) on the cells.
If $R$ is one such Voronoi cell, then set $Q:=R\cap C$. Then indeed
$$C=\bigcup_{g\in G} g\cdot Q,$$
and, while not explicitly requested, we also have that $g\cdot G$ and $g'\cdot G$ meet only in a proper face whenever $g\not=g'$.
$Q$ is the intersection of a rational cone with a rational polytope, thus, itself rational.
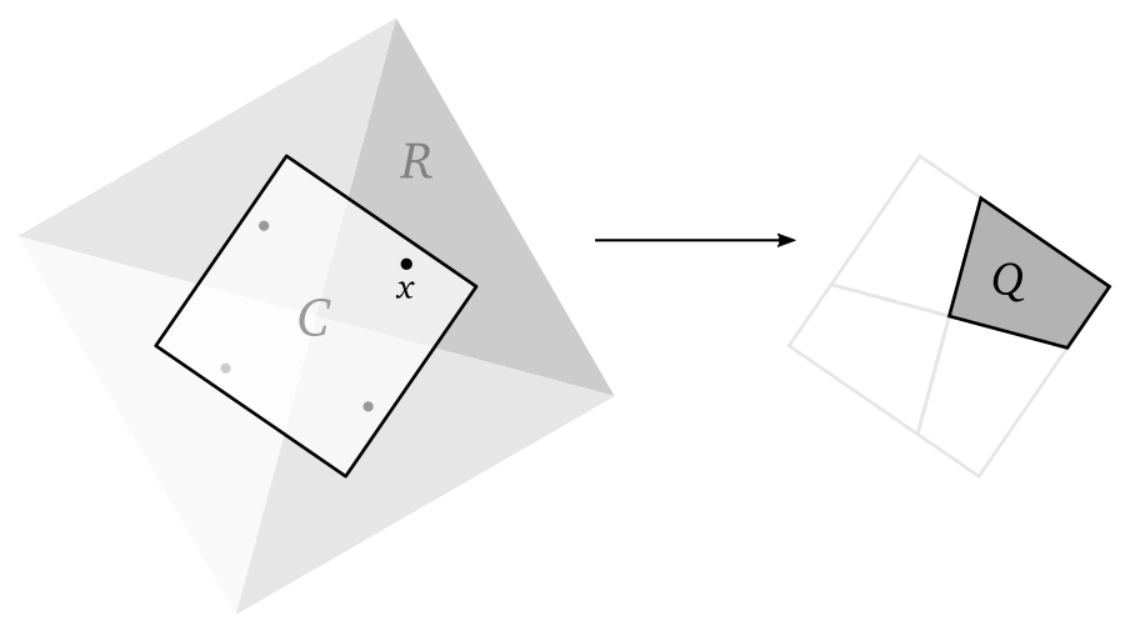
The image shows an example where $G$ is the cyclic group generated by a $90^\circ$ rotation.
