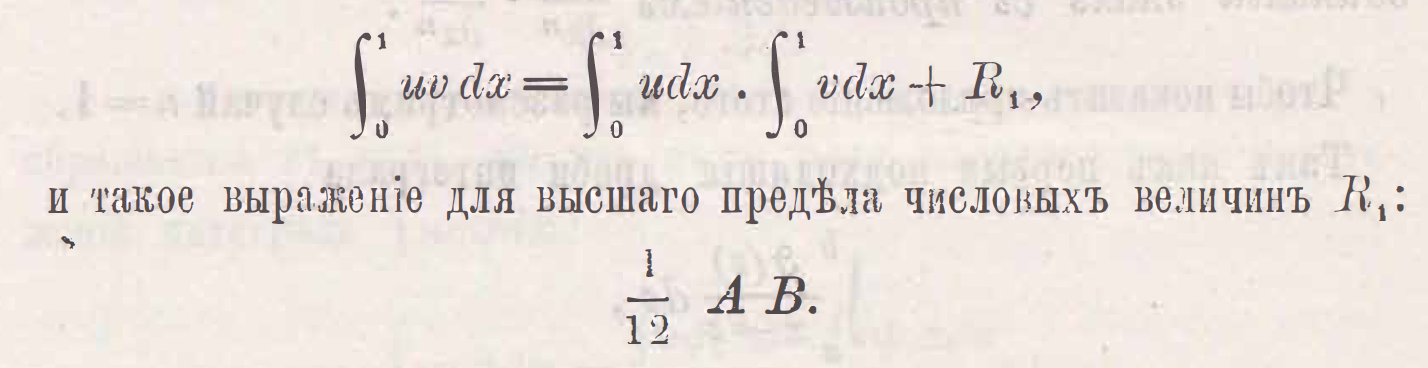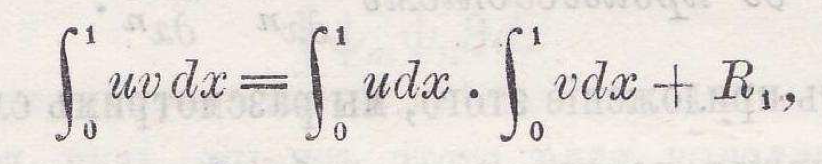The source for the inequality is
P.L. Chebyshev, On approximate expressions of some integrals in terms of others, taken within the same limits, Proc. Math. Soc. Kharkov 2, 93–98 (1882).
This article is online here, in Russian. The relevant equationtext is copied below, with the statement that $R_1=AB/12$ and $A,B$ being the largest of the derivatives of $du/dx$ andwhich presumably states $dv/dx$$R_1>0$, hence $R_1\geq 0$ for monotone $f,g$hopefully someone who reads Russian can provide a translation.
Someone who reads Russian will correct me if I misinterpreted Google translate suggests that $R_1=AB/12$ with $A,B$ the textmaximum of the derivatives of $du/dx$, $dv/dx$, which as noted by Emil Jeřábek cannot be quite correct.