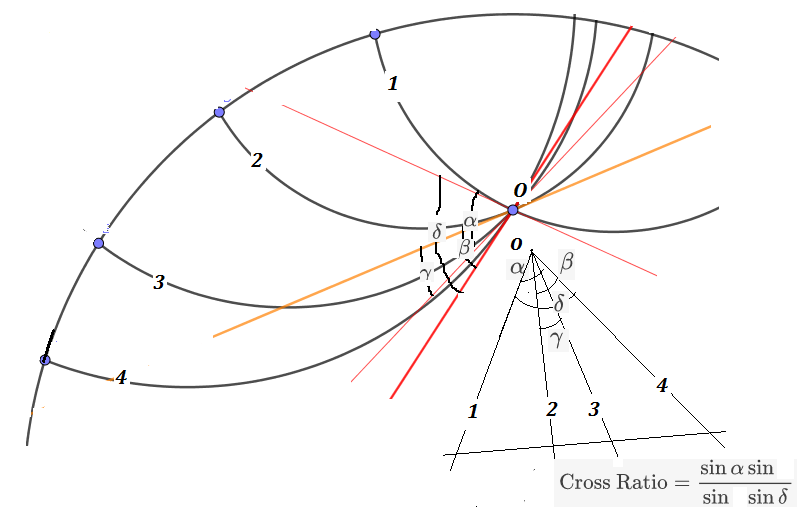
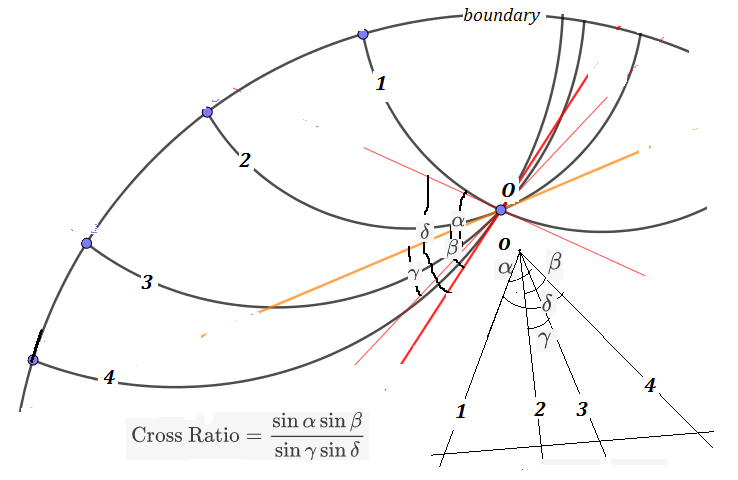
In the rough sketch four concurrent lines are drawn in the Poincaré disk model and in the Euclidean model.
If same angles $ (\alpha,\beta,\gamma,\delta) $ are madeenclosed at respective points of concurrency $(o,O)$ as shown between lines $(1,2,3,4)$ in either model, then does the same trig definition of Cross Ratio hold good?
If that is so, and if the same three adjacent angles are given then is it correct to say they have the same Cross Ratio in euclidean and hyperbolic geometries. Is this correct ?
I need your help, appreciate your comments.