Up to scale change and multiplication by a constant, the function is
$\frac 1 {x^2} \sin^2 x \cos x \sin 2x = \frac {\sin x} x \frac {\sin x} x \cos x \sin 2x$
We shall use the convolution theorem, i.e. that the Fourier transform of $f(x)g(x)$ is $\frac 1 {2\pi} \hat f(\nu) * \hat g(\nu)$.
The Fourier transform of $\frac {\sin x} x$ is $\pi \cdot \text{rect}(\nu/2)$. The transform of $\frac {\sin^2 \nu} {\nu^2}$$\frac {\sin^2 x} {x^2}$ is thus $1/2\pi$ times the convolution of two such functions, which I will denote by $T(\nu)$, because it looks like a triangle. ;-)
The Fourier transform of $\cos(x)$ is $\pi \delta(\nu-1)+\pi \delta(\nu+1)$. For $\sin 2x$, it is $-i\pi \delta(\nu-2)+i\pi \delta(\nu+2)$. Their convolution multiplied by $1/2\pi$ is $\frac {i\pi} 2(-\delta(\nu-1)+\delta(\nu+1)-\delta(\nu-3)+\delta(\nu+3))$. The same result could be obtained by expanding into complex exponentials and then Fourier-transforming.
Finally, the transform of the original function is the convolution of these two functions divided by $2\pi$, which is $\frac i 4 (-T(x-3)-T(x-1)+T(x+1)+T(x+3))$. The function is purely imaginary, as it should be for an odd function of $x$.
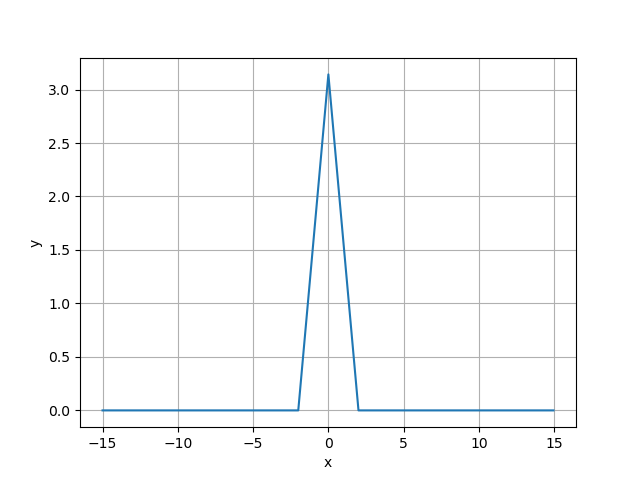 This is the triangle function, $T(x)$.
This is the triangle function, $T(x)$.
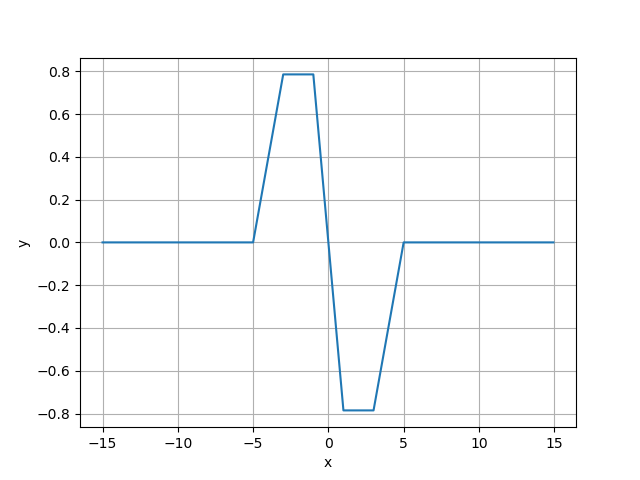 And this is the imaginary part of the Fourier transform (the real part is obviously zero).
And this is the imaginary part of the Fourier transform (the real part is obviously zero).


