For generic $F$ the answer is probably yes, but for certain $F$ and certain choices of $\epsilon_i$ the answer is no, not necessarily.

Here is an example with n=2 and $M$ the Klein bottle. We start with F being a standard projection of the Klein bottle into the plane. This is easy to visualize by googling for images of these glass Klein bottles that are everywhere.

With the standard projection we get that $W_F$ is an embedded circle and $M_F$ has just one component. So in that case the integral will be non-zero for each of the two possible orientations.

However now we are going to deform F slightly. We can imagine that our glass Klein bottle has a lump/boil/blister and that it is really quite large. This gives use a new projection from the Klein bottle to the plane. The image looks something like this:

The new $W_F$ is the inverse image of the red curve. It is now two circles. $M_F$ now has two components, one of which is a disk. Topologically we have taken the old $M_F$ and removed a disk.

When we integrate $\omega_F$ over these two pieces we will get numbers and by expanding the "boil" we can make it so that these numbers are exactly matched in magnitude. Then with the correct choice of $\epsilon_i$'s we can make these factors contribute oppositely so that for this particular $F$ and this particular choice of $\epsilon_i$'s the net integral vanishes.

I think it is always possible to do a similar trick/deformation.

For certain $F$ and $\epsilon_i$ the answer is no. But it is probably yes for generic choices.

Here is an example with n=2 and $M$ the Klein bottle. We start with F being a standard projection of the Klein bottle into the plane. This is easy to visualize by googling for images of these glass Klein bottles that are everywhere.

With the standard projection we get that $W_F$ is an embedded circle and $M_F$ has just one component. So in that case the integral will be non-zero for each of the two possible orientations.

However now we are going to deform F slightly. We can imagine that our glass Klein bottle has a lump/wort/blister and that it is really quite large. This gives use a new projection from the Klein bottle to the plane. The image looks something like this:

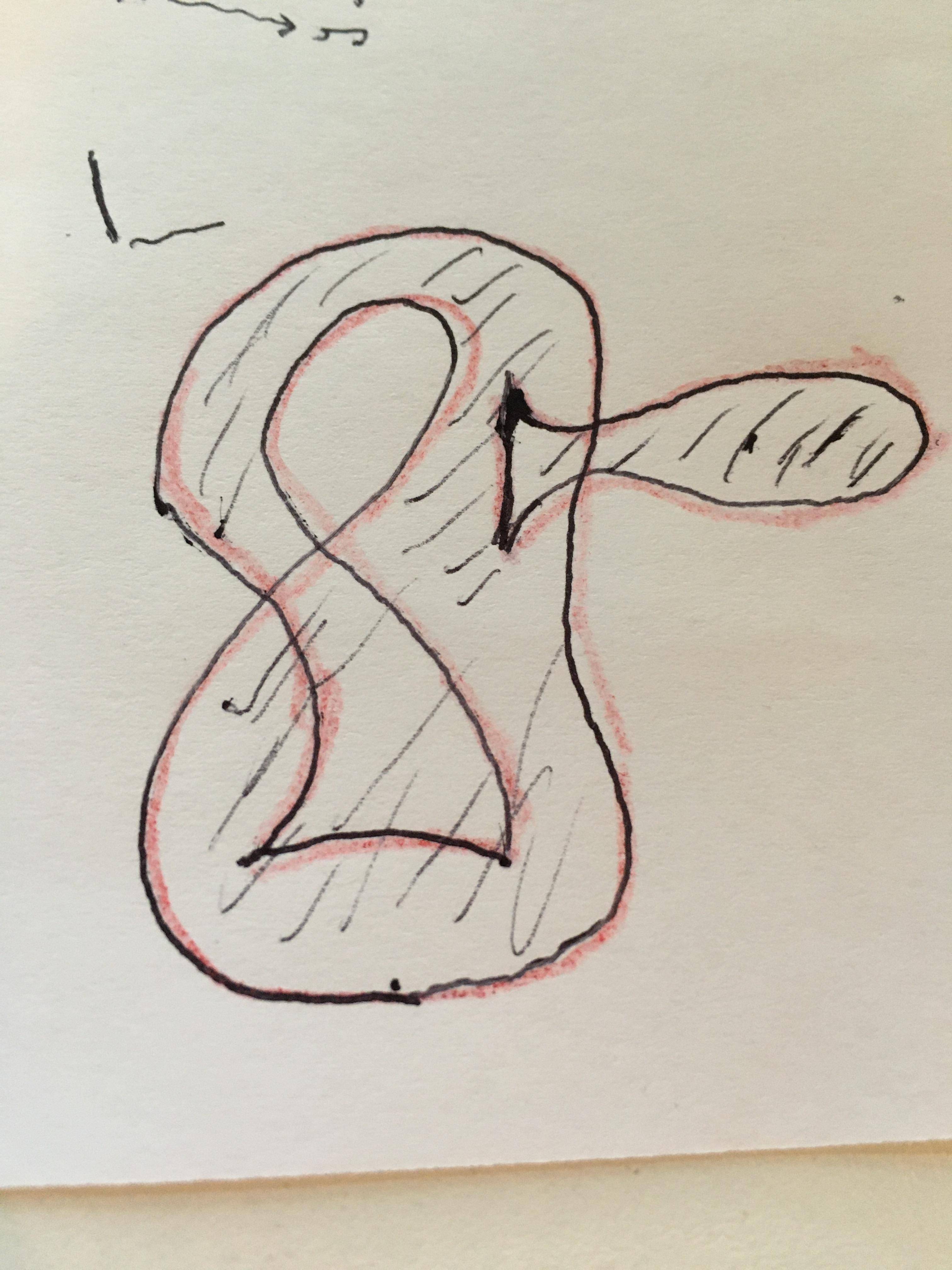
The new $W_F$ is the inverse image of the red curve. It is now two circles. $M_F$ now has two components, one of which is a disk. Topologically we have taken the old $M_F$ and removed a disk. Let me call these regions $M_0$ and $M_1$ (the disk) following the notation of the OP.

When we integrate $\omega_F$ over these two pieces we will get numbers which are essentially the areas of the images of these regions under $F$ (with signs depending on the $\epsilon_i$/$\textrm{or}_i$). Now we can imagine expanding the "wort" - making its image in the plane larger. This will change the magnitude of the integral over the two pieces $M_0$ and $M_1$. My original idea was that we could make it so that these numbers are exactly matched in magnitude. Then with the correct choice of $\epsilon_i$'s we can make these factors contribute oppositely so that for this particular $F$ and this particular choice of $\epsilon_i$'s the net integral vanishes.

However that does not quite work. The reason is the the "wort" is split into two halves. The, say, lower half contributes to $M_1$ (it essentially is the disk) but the upper half contributes to $M_0$. When we deform the "wort" the upper and lower half are deformed in matching ways. So we can't quite ensure that the magnitudes of the integrals become equal.

However, now what we can do is add a second "wort" onto the first. We make sure the second "wort" emerges from the lower half (the $M_1$ half). The corresponding image in the plane looks something like this:

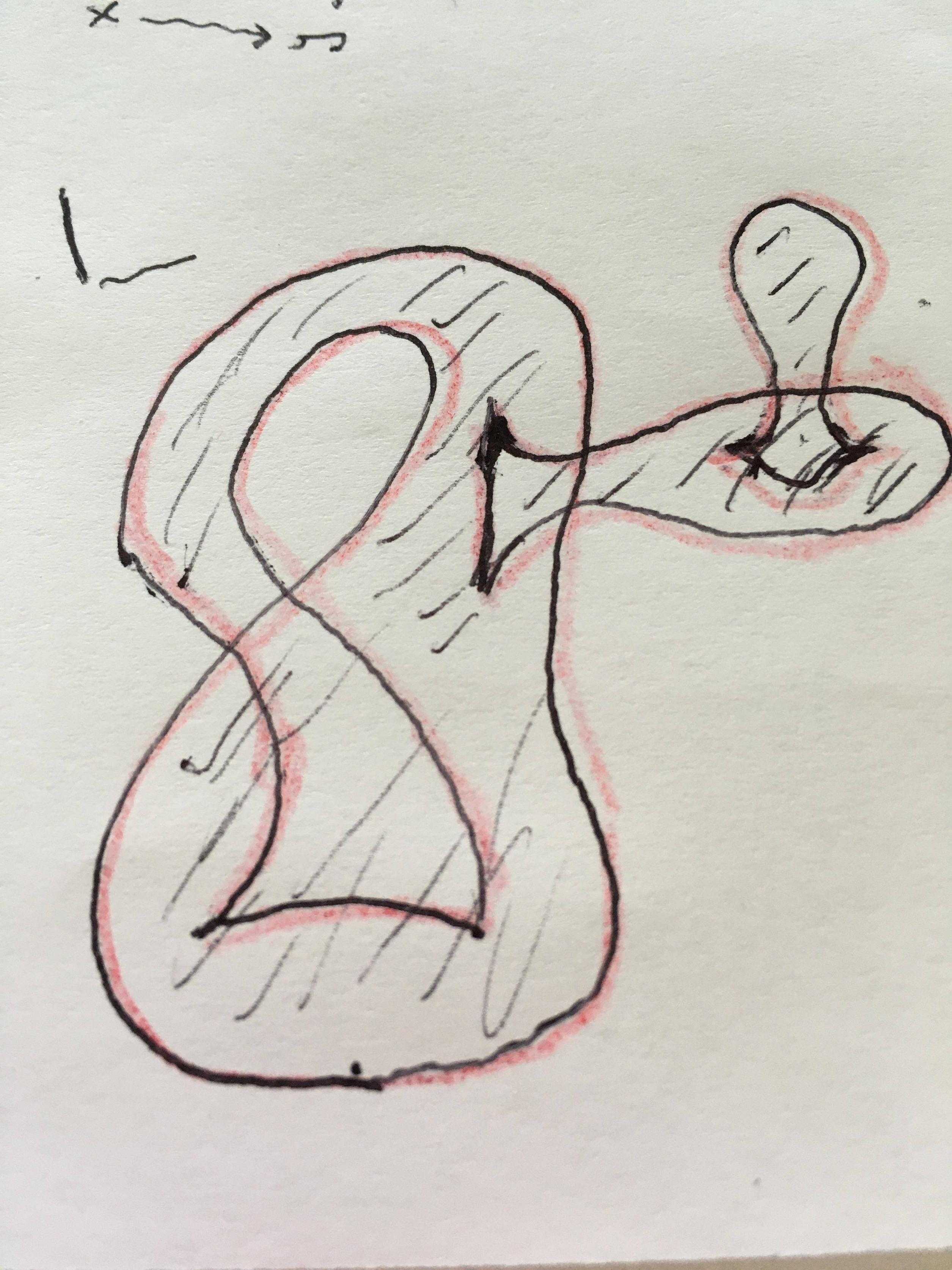
Now $W_F$ is three circles, and $M_F$ has a new component $M_2$ which is a disc which has been cut out of the old $M_1$. When we enlarge the new "wort" it again increases the magnitude of the integral on two regions, but now the two regions involved are $M_1$ and $M_2$.

So now we can adjust the size of the new wort so that the magnitude of the integral on $M_0$ exactly matches the sum of the magnitudes on $M_1$ and $M_2$. Now with appropriate choices of $\epsilon_i$'s, the total integral over $M_F$ will cancel.

I think it is always possible to do a similar trick/deformation.