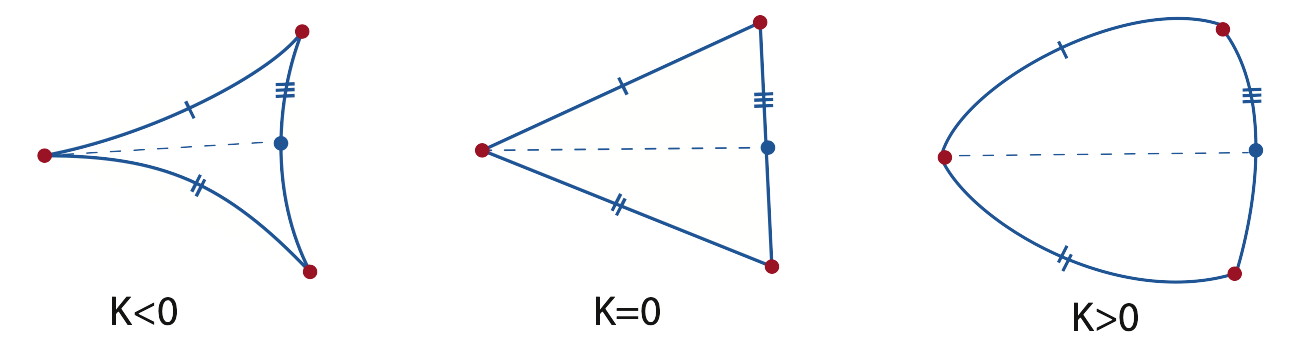
The best way I know to illustrate the notion of curvature is via Toponogov's theorem. We can compare any (geodesic) triangle in a Riemannian manifold $M$ with one with the same edge lengths in Euclidean plane $R^2$. The (sectional) curvature of $M$ is positive (resp. negative) provided that all its triangles are fatter (resp. thinner) than the comparison triangle. More precisely, this means that the distance between each vertex and the midpoint of the opposite side is bigger (resp. smaller) than the corresponding distance in the comparison triangle.