I'm fond of the little problem posed in the beginning of this Quanta article: take $n$ generic points on a circle and draw the complete graph between those points. Into how many regions do the edges cut the circle?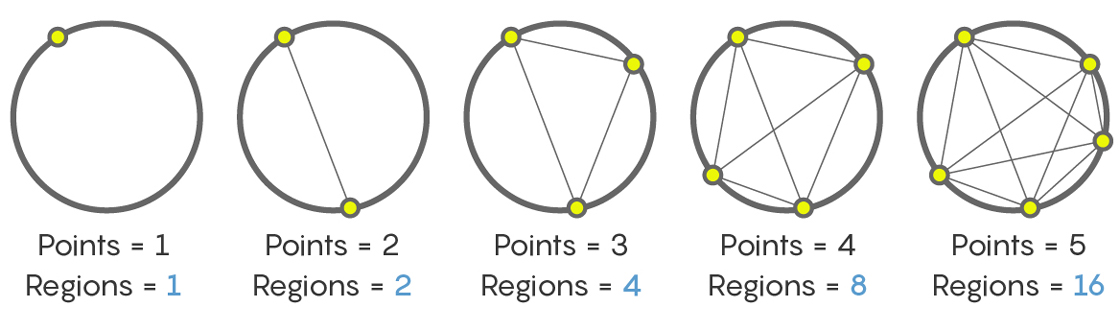

You can compute a few examples and are quickly led to conjecture that $n$ points yield $2^{n-1}$ regions. But the next example falls short: $n=6$ points yield $31$ regions. This is a nice lesson in the surprises that math has to offer. And the actual solution to the problem can be found using a bit of combinatorial reasoning and Euler's formula, which shows how you can rope in different areas of math to solve an apparently simple problem.