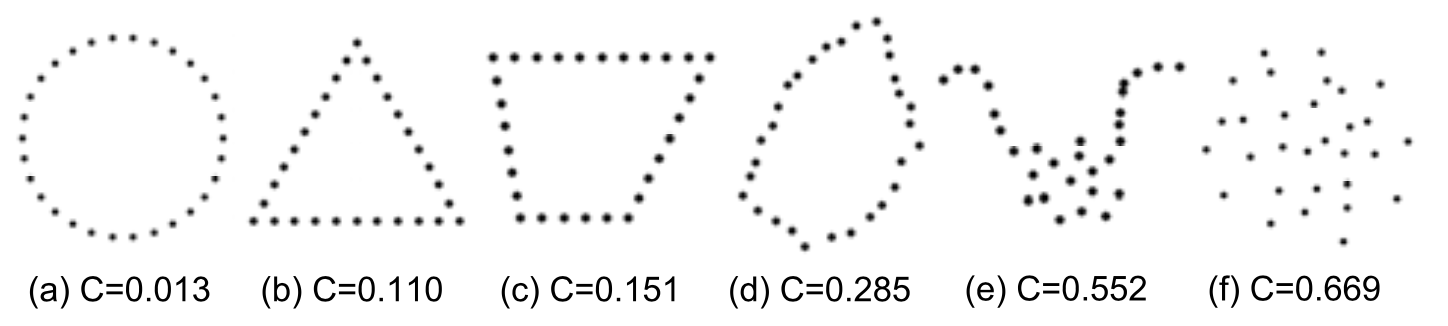
This problem is addressed in Estimating Complexity of 2D Shapes (2005). The notion of "complexity" seems to agree at least qualitatively with what the OP calls "uniformity". The complexity measure $C$ of a 2D shape is quantified by the combination of three criteria – (a) entropy of the global distance distribution, (b) entropy of local angle distribution, (c) shape randomness. The figure below shows results for 6 shapes, digitizeddiscretized by a set of points. Small $C$ means low complexity.