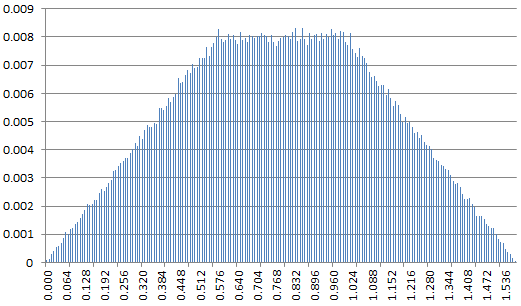
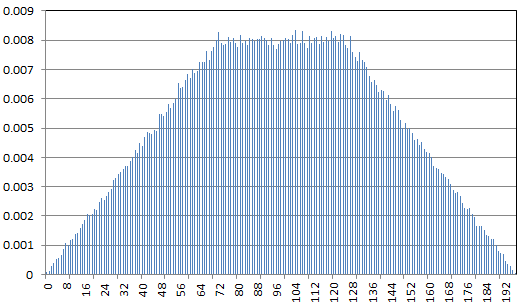
Here $b_1, b_2>0$ are integers. I investigated the sequence $Y_k=X_{3k}$, which has far more communal planes, and thus more useful to build a random generator. Of course, choosing large values for $b_1,b_2$ will further drastically improve the generator by adding a lot more planes. I suggest choosing values larger than (say) $2^{30}$ for $b_1,b_2$.
More compact version and exact formula for number of communal planes is now provided
Vincent Granville
- 3.1k
- 8
- 21
I discovered a general formula for the equation of the parallel communal planes, for positive $b_1,b_2>1$ (I don't have a proof yet)
Vincent Granville
- 3.1k
- 8
- 21
Vincent Granville
- 3.1k
- 8
- 21