I think the statement in the OP that $W_2(a)$ and $W_3(a)$ remain bounded when $a\rightarrow 0$ is mistaken, so that there is no inconsistency with the Mathematica result.
The three roots of $(w+1)^3+a^3=0$ are $$w_1= -a-1,\;\; w_2= \tfrac{1}{2} \left(-i \sqrt{3} a+a-2\right),\;\;w_3= \tfrac{1}{2} \left(i \sqrt{3} a+a-2\right).$$ Then the denominator $(w+1)^2$ vanishes for all three roots when $a\rightarrow 0$, while the numerator remains finite (equal to $-\gamma_{\rm Euler}$).
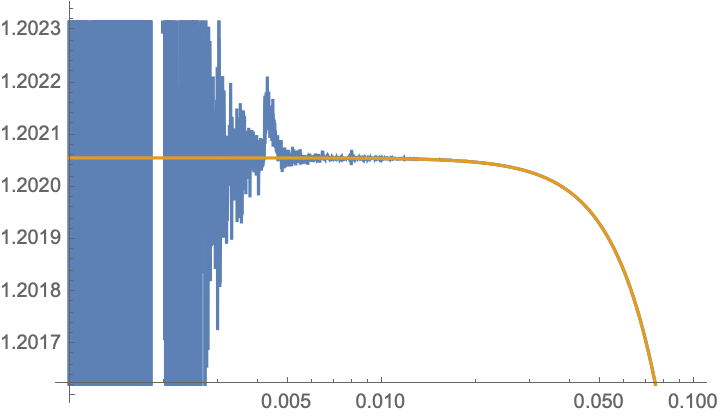
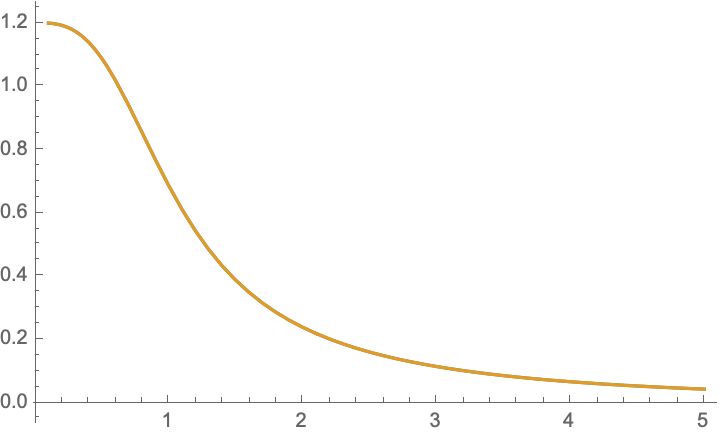
And indeed, a numerical check suggets that the Mathematica output is actually correct, and the erroneous numerical result for small $a$ is a numerical instability in the computation of the digamma function. See these two plots that compare the digamma expression (blue) with a numerical evaluation of the sum (gold), as a function of $a$. For $a\gtrsim 0.01$ the two answers are nearly indistinguishable.