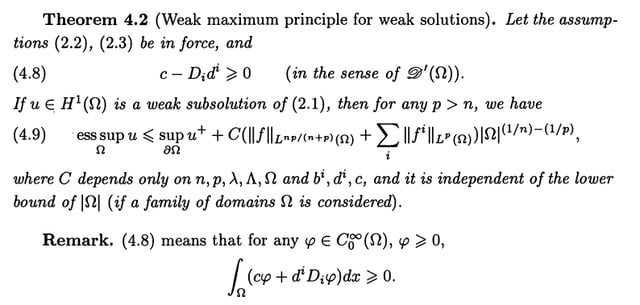
I am learning about elliptic PDEs using the book by Chen & Wu, especially on the maximum principle. The author uses the De Giorgi iteration technique to establish the weak maximum principle for elliptic operators under some conditions. I attached the statement here, and you can also see the proof in this link.
Before asking my question, I will describe the scheme of the proof briefly. As the main lemma of the De Giorgi iteration, the following is presented.
Lemma. Suppose $\varphi(t)$ is a nonnegative decreasing function on $[k_0, \infty)$ with $$ \varphi(h) \leq \frac{C}{(h-k)^\alpha} \varphi(k)^\beta$$ for $h>k\geq k_0$, where $\alpha>0, \beta>1$. Then, for $$d = C^{1 /\alpha} \left[ \varphi(k_0)\right]^{(\beta -1)/{\alpha}} 2^{\beta / (\beta -1)},$$ we have $$ \varphi(k_0 + d) = 0.$$
Then, we want to appliedapply this lemma to the measure of the sets $$ A(k) = \left\lbrace x \in \Omega \ \vert \ u(x) >k \right\rbrace, \quad k \in \mathbb R$$ in order to obtain thean upper bound of the essential supremum of $u$ on $\Omega$. After some estimation and using the lemma, we can get the following result.
Result. Let $\tilde C $ be the embedding constant of the Sobolev embedding of $W^{1,2}_0 (\Omega)$. Suppose $k_0 \geq l := \sup_{\partial \Omega} u^+$ satisfies $${\tilde C}^2 \left\vert A(k_0) \right\vert^{2/{n}} \leq \frac{1}{2}.$$ Then, $$\DeclareMathOperator{\esssup}{\mathrm ess \, sup} \esssup_{\Omega} \leq k_0 + CF_0 \lvert \Omega\rvert^{(1/n) - (1/p)} =: k_0 + C \tilde{F}_0,$$ where $F_0 = \frac{1}{\lambda} \left( \sum_i \lVert f^i \rVert_{L^p} + \lVert f \rVert_{L^{p_*}} \right)$ and $p_* = np/(n+p)$.
To get $k_0$, we can first use the Chebyshev inequality on $u$. Then, we obtain some $k_0$ such that $k_0 \leq \sup_{\partial \Omega} u^+ + C \lVert u \rVert_{L^2}$, but this only guarantees the essential boundedness of $u$ on $\Omega$. Thus, we need to estimate further.
In order to obtain a better choice of $k_0$, the following test function is chosen: for $v = (u-l)^+$, $$ \varphi = \frac{v}{M+ \epsilon + \tilde{F}_0 - v} \in W^{1,2}_0(\Omega),$$ where $M = \esssup_{\Omega} u - l$. This gives a better estimate on $\left\vert A(k) \right\vert$ than the estimate by the Chebyshev inequality: for $l<k<\esssup_{\Omega} u$, $$ \left\vert A(k) \right\vert^{1/2^*} \log \frac{M+ \epsilon + \tilde{F}_0}{M+ \epsilon + \tilde{F}_0 - (k-l)} \leq \textrm{constant},$$ where $2^*$ is the Sobolev conjugate of $2$.
Now I can tell my question: is there some intuition behind choosing that test function? I am trying to find some reason for that choice, but I don't figure it out currently. I only understand that such a choice provides a better estimate.
I heard that such a kind of a test function is frequently useful, and in fact it is often used. By searching some references, I found that N. Trudinger also used the same type of the test function in 1973's and 1977's papers. I think there is some hint in the estimate procedure, but I don't grasp any idea from that.
Could you give me some intuition about that? Also, I would like to ask what way of thinking (or algorithm) is useful when choosing a test function in an estimation procedure. Thanks!
Addition: I think I should mention my opinion on why the last estimate on $\lvert A(k)\rvert$ is better. First, it does not involve the $L^2$-norm of $u$ anymore. It exactly contains our desired quantities: $\esssup_{\Omega} u$, $\sup_{\partial \Omega}u^+$ and $F_0$. In addition to this, by its form, we can easily relate $k$ and the other quantities as in the proof from the book. In this context, I can change my question to be more specific: what intuition does make somebody expect to get an estimate with those nice features?
It could be just obtained by some trials and errors. I fully understand that it would be possible that there is no critical insight. But then, what would be the starting point of this strategy?
I might be just complicating a simple stuff. I could just accept this part of the proof as a technique or machinery. However, I am really curious about what is a source of it. That is why I posted this question.