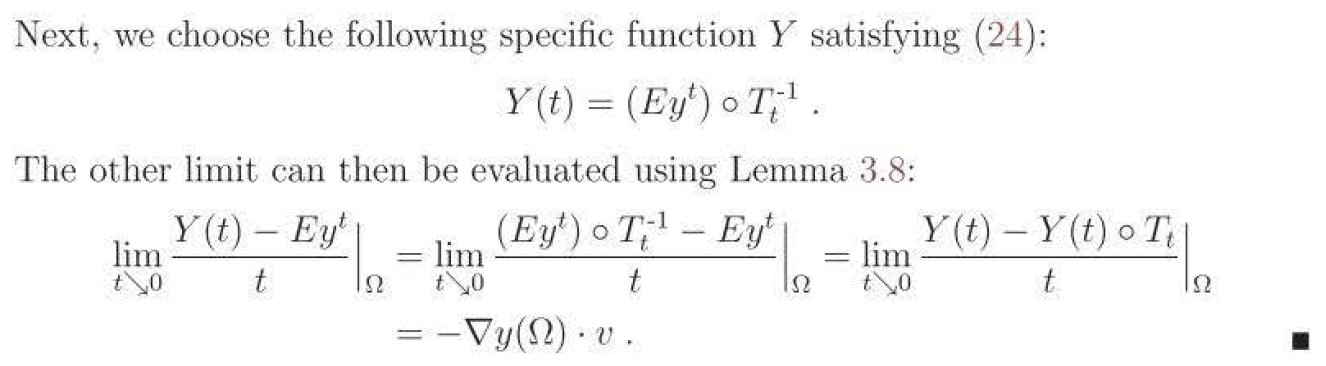I've started reading about shape optimization. Most of the concepts I've encountered so far (such as the shape derivatives of domain and boundary integrals and the corresponding) seem to be complex, but turned out to be quite simple. However, I really struggle to wrap my head around the different notions of "derivatives" for a "shape-dependent" function $y$.
The setting is as follows: Let
- $d\in\mathbb N$;
- $D\subseteq\mathbb R^d$ be open and $\mathcal A\subseteq 2^D$ with $D\in\mathcal A$;
- $E_\Omega\subseteq\mathbb R^{\Omega}$ be a $\mathbb R$-Banach space for $\Omega\in\mathcal A$ and $E:=\bigcup_{\Omega\in\mathcal A}E_\Omega$;
- $y:\mathcal A\to E$ with $$y(\Omega)\in E_\Omega\;\;\;\text{for all }\Omega\in\mathcal A;\tag1$$
- $\tau>0$ and, $T_t:D\to D$$T_t$ be a $C^1$-diffeomorphism from $U$ onto an open subset of $\mathbb R^d$ for $t\in[0,\infty);$$t\in[0,\infty)$ and $$V:=\bigcup_{t\in[0,\:\tau)}T_t(D);$$
- $v:[0,\tau)\times D\to\mathbb R^d$$v:[0,\tau)\times V\to\mathbb R^d$ be differentiable in the second argument with $$v\left(t,T_t(x)\right)=\frac{\partial T}{\partial t}(t,x)\;\;\;\text{for all }(t,x)\in[0,\tau)\times D;\tag2$$
- $\Omega\in\mathcal A$ and $\Omega_t:=T_t(\Omega)$ for $t\in[0,\tau)$.
Now the "shape derivative* is defined as follows:
Definition 1 (shape derivative) Let $Y:[0,\tau)\to E_d$ with $$\left.Y(t)\right|_{\Omega_t}=y(\Omega_t)\;\;\;\text{for all }t\in[0,\tau).$$ Then $y$ is called shape differentiable at $\Omega$ in direction $v$ if $Y$ is Fréchet differentiable at $0$. In that case, $$y'(\Omega;v):=\left.Y'(0)\right|_{\Omega}\tag4.$$ (Please note that we most probably need to assume a certain regularity (at least continuity) of the time-dependence of $Y$ (and most probably of $T$ as well). I've omitted them, cause it's part of my question what we need to assume precisely.)
The second definition is given by the "material derivative*:
Definition 2 (material derivative) $\dot y(\Omega;v)\in E_\Omega$ is called **material derivative of $y$ at $\Omega$ in direciont$ v$ if $$y(\Omega_t)\circ\left.T_t\right|_{\Omega}\in E_\Omega\;\;\;\text{for all }t\in[0,\tau)\tag5$$ and $$[0,\tau)\to E_\Omega\;,\;\;\;t\mapsto y(\Omega_t)\circ\left.T_t\right|_{\Omega}\tag6$$ is Fréchet differentiable at $0$ with derivative equal to $\dot y(\Omega;v)\in E_\Omega$, i.e. $$\frac{y(\Omega_t)\circ\left.T_t\right|_{\Omega}-y(\Omega)}t\xrightarrow{t\to0+}\dot y(\Omega;v)\tag7.$$
Question 1: What do we need to assume in order to show that $(3)$ is well-defined, i.e. independent of the choice of $Y$?
Question 2: How can we relate the shape and material derivative?
I guess we need to assume that there is a continuous linear $$\iota_A:E_A\to E_D$$ for all $A\in\mathcal A$. Let $$y_t:=y(\Omega_t)\circ\left.T_t\right|_{\Omega}\;\;\;\text{for }t\in[0,\tau).$$ Then we could write $$\frac{Y(t)-Y(0)}t=\frac{Y(t)-\iota_\Omega y_t}t+\frac{\iota_\Omega y_t-Y(0)}t\;\;\;\text{for all }t\in(0,\tau)\tag8.$$ If the answer to question 1 is positive, then we could assume $$Y(t)=\iota_{\Omega_t}y(\Omega_t)\;\;\;\text{for all }t\in[0,\tau)\tag9$$ and, assuming $y$ has a material derivative at $\Omega$ in direction $v$, we could conclude $$\frac{\iota_\Omega y_t-Y(0)}t=\iota_\Omega\frac{y_t-y(\Omega)}t\xrightarrow{t\to0+}\iota_\Omega\dot y(\Omega;v)\tag{10}.$$
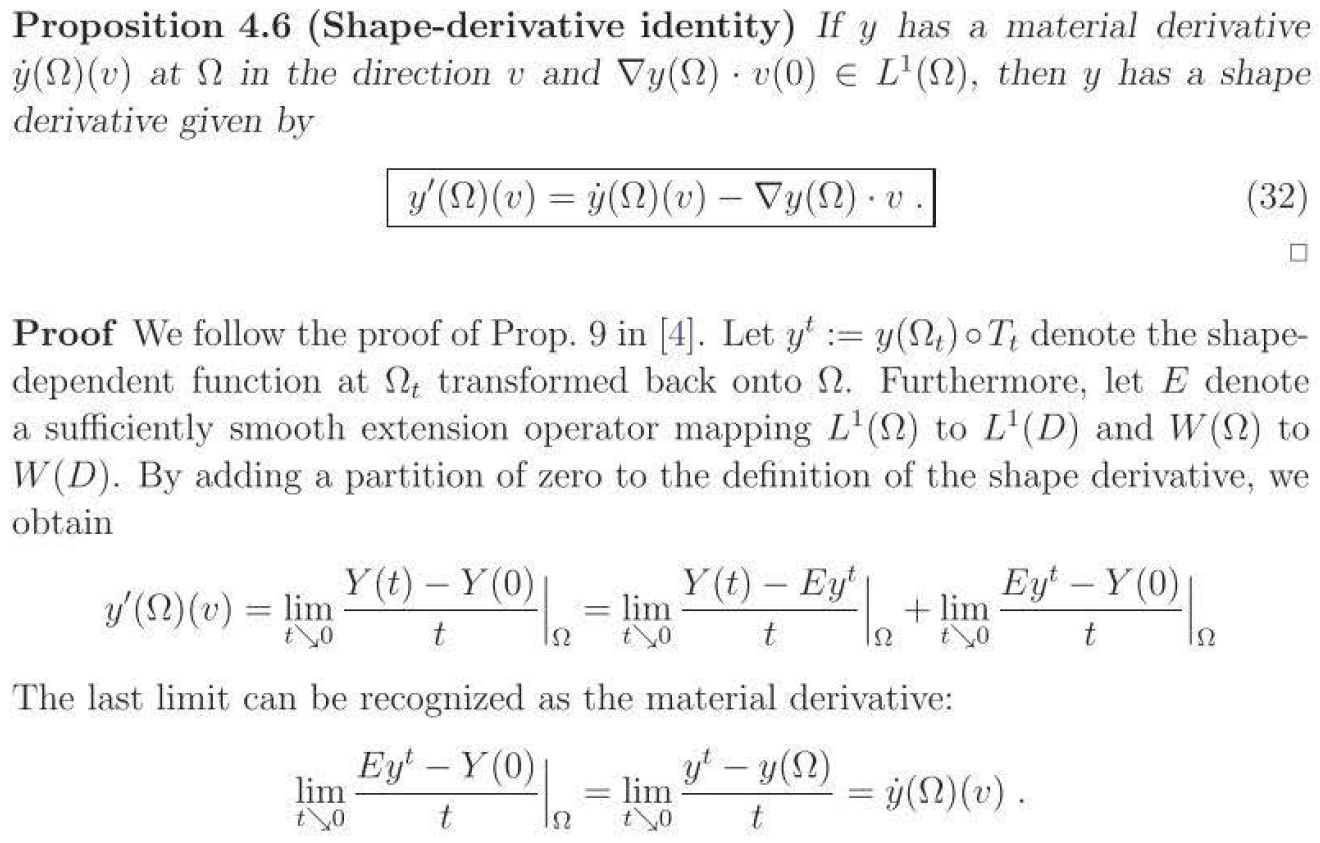
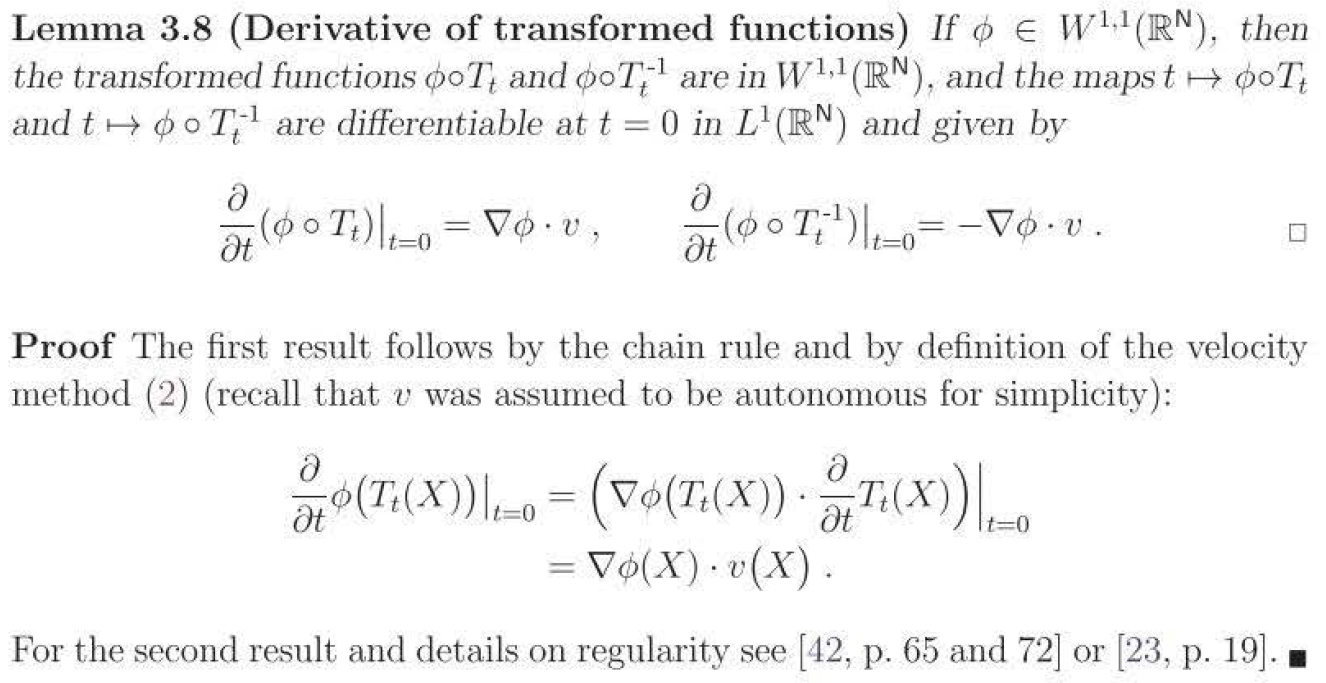
Note that there is a proof of the "shape-derivative identity" in this paper, but I think their proof is missing rigor and they seem to assume $E_\Omega$ is a closed subspace of $L^1(\Omega)$: