Please don't close this question. Because there is simple configuration with 57 vote up, and don't close. Why you vote up that question and You vote to close this question?
A problem I posed at here since 2014 but no solution:
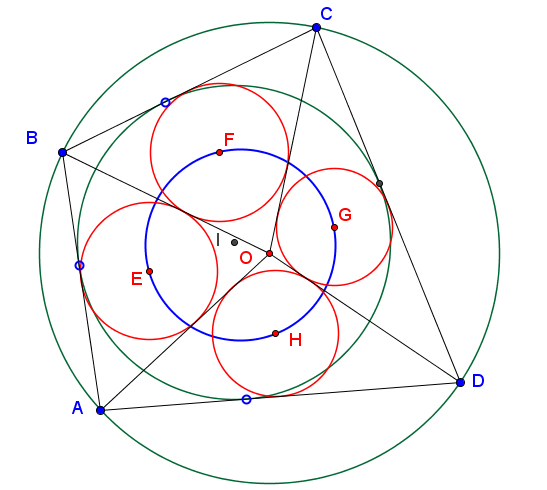
Let $ABCD$ be a bicentric quadrilateral, $O$ is center of circle $(ABCD)$. Then Incenter of four triangles $OAB,OBC,OCD,ODA$ lie on a circle.
My question: Could You give a your solution for problem above.
The problem like Bradley’s conjecture. You can see Bradley’s conjecture at here and page 73, here