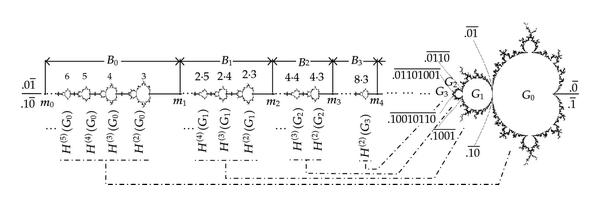
If you change the form of your map to $z_{n+1} = z_n^2 + c $ ( conjugation) and take only real values of c ( real slice of Mandelbrot set) then you will find the answers in papers by G. PastorG. Pastor, M. Romera. Here is for example : Calculation of the Structure of a Shrub in the Mandelbrot Set
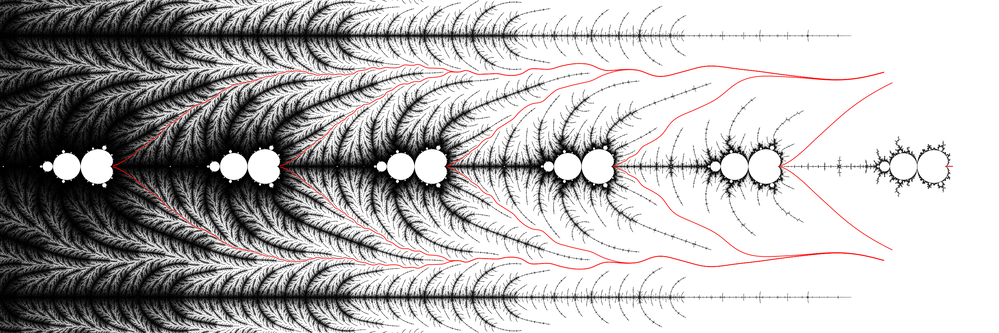
The part from 0 to Feigenbaum point in your map is a periodic region where period doubling cascade occurs. In c plane it is from 0.25 to F
The part from F to 4 in your map is a Mandelbrot set antenna. It's structure is described in that paper :
Look also for:
"Many questions concerning (discrete) dynamical systems are of a number theoretic or combinatorial nature." Christian Krattenthaler
HTH