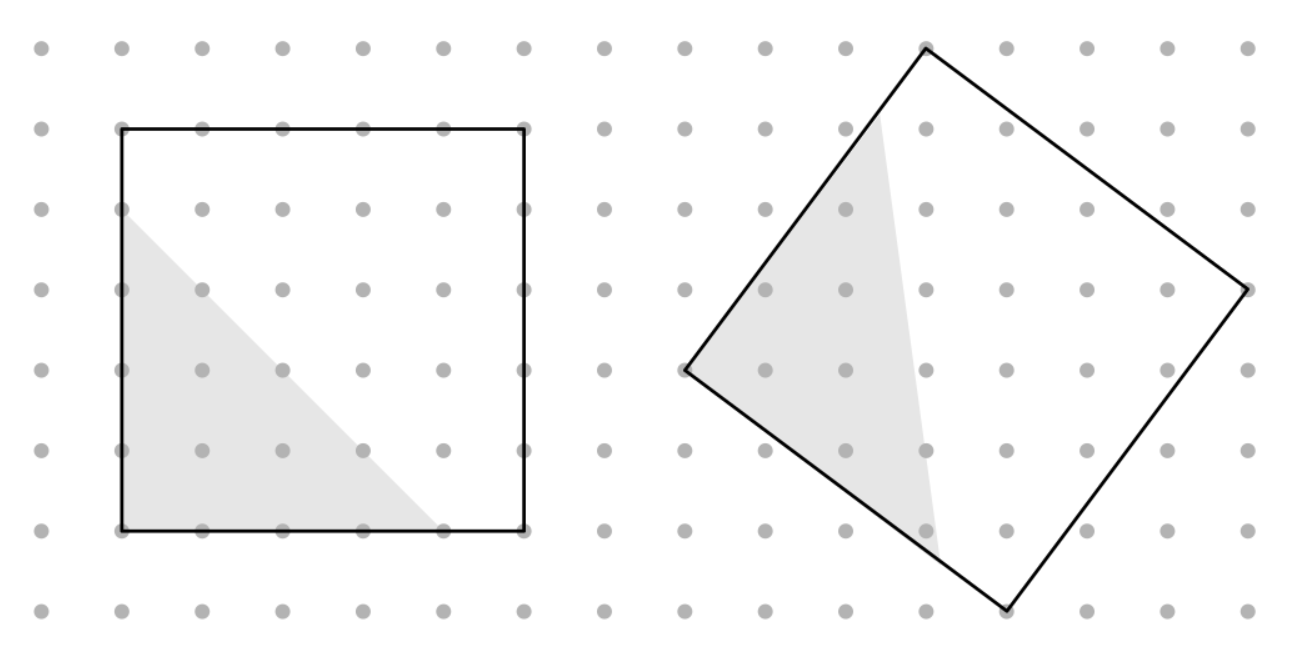
The answer to your first question is No.
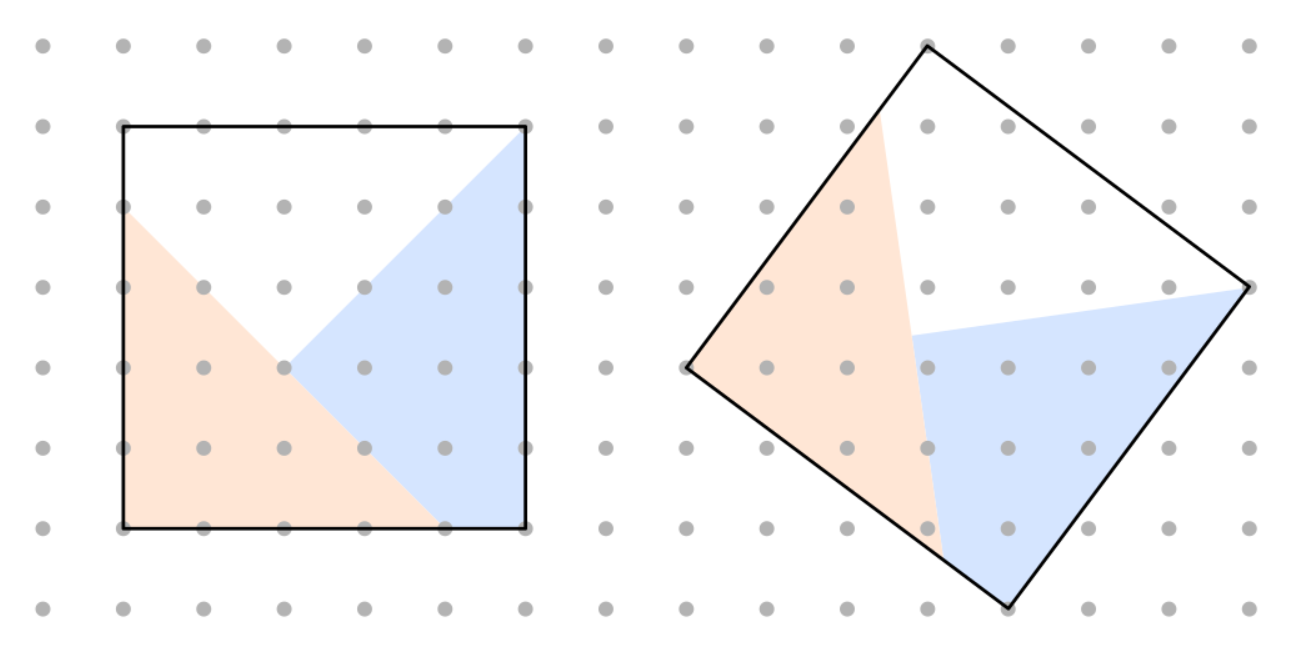
Consider the 5x5 square as a lattice polytope in two different ways as seen above. Each tiling of one of these sqares gives a tiling of the other. But you can choose the tiles in such a way, so that they are lattice polytope in only one of the squares.
So the right square is tiled with quasi-lattice polytopes (as seen in the left square) that are not lattice polytopes.