If you want your "reshaping" transformation function to be monotonic, then the answer is no.
Indeed, suppose that $X_j\sim\text{gamma}(a_j,1)$ for $j=1,2$. Let $F_j$ be the cdf of $X_j$.
Suppose that $X_2$ equals $f(X_1)$ in distribution for some continuous strictly increasing function $f$. Then for all real $x_2$ $$F_2(x_2)=P(X_2\le x_2)=P(f(X_1)\le x_2)=P(X_1\le f^{-1}(x_2)) =F_1(f^{-1}(x_2)),$$ so that $F_2=F_1\circ f^{-1}$ and hence necessarily $$f=F_2^{-1}\circ F_1,$$ just as you had it.
Similarly, if $X_2$ equals $f(X_1)$ in distribution for some continuous strictly decreasing function $f$, then $$f=F_2^{-1}\circ G_1,$$ where $G_1:=1-F_1$.
However, in the case when $a_1=a_2+1$, there is a curious somewhat related equidistribution phenomenon (that note can be read online for free).
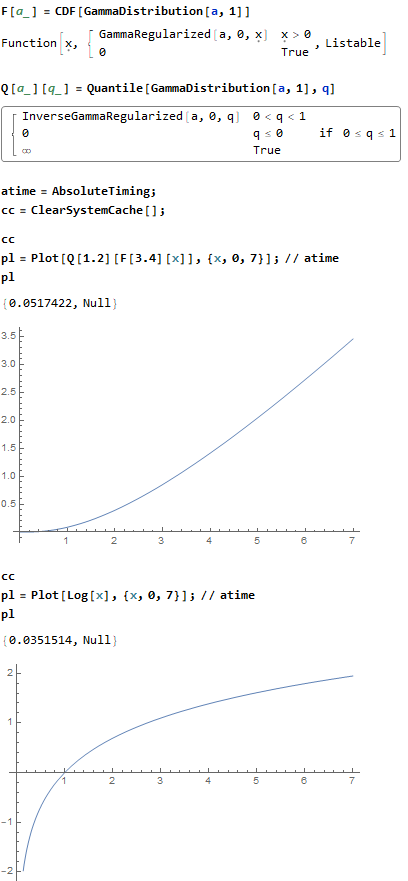
I don't think that the expression $F_2^{-1}\circ F_1$ is hard to deal with. E.g., Mathematica produces the graph $\{(x,(F_2^{-1}\circ F_1)(x))\colon0<x<7\}$ for $a_1=1.2$ and $a_2=3.4$ in about $0.052$ sec; for a comparison, it produces the graph $\{(x,\ln x)\colon0<x<7\}$ in about $0.035$ sec. Also, I think it is not hard to deal with $F_2^{-1}\circ F_1$ analytically. Here is the graph production: